- #1
Math Amateur
Gold Member
MHB
- 3,996
- 48
I am reading Matej Bresar's book, "Introduction to Noncommutative Algebra" and am currently focussed on Chapter 1: Finite Dimensional Division Algebras ... ...
I need help with another aspect of the proof of Lemma 1.1 ... ...
Lemma 1.1 reads as follows:
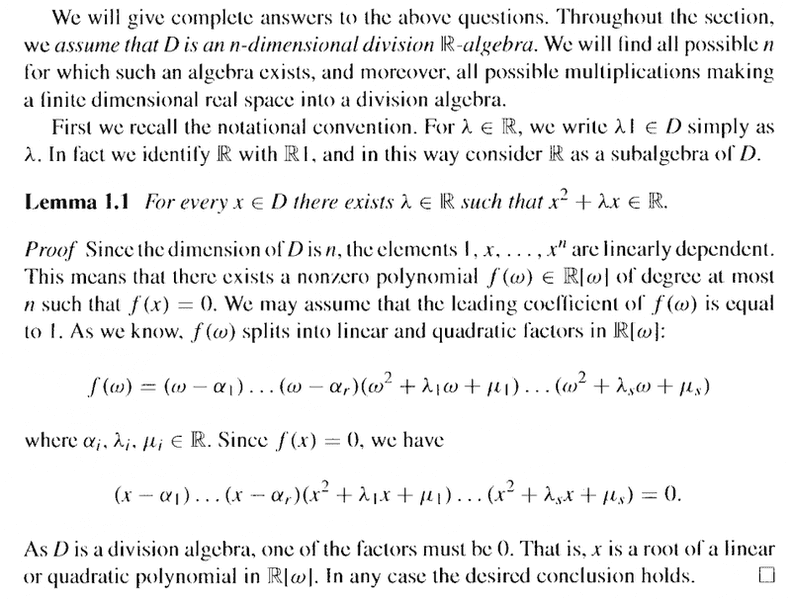
My questions regarding Bresar's proof above are as follows:Question 1
In the above text from Bresar, in the proof of Lemma 1.1 we read the following:
"... ... As we know, ##f( \omega )## splits into linear and quadratic factors in ##\mathbb{R} [ \omega ]## ... ..."
My question is ... how exactly do we know that ##f( \omega )## splits into linear and quadratic factors in ##\mathbb{R} [ \omega ]## ... can someone please explain this fact ... ...
Question 2
In the above text from Bresar, in the proof of Lemma 1.1 we read the following:
" ... ... Since ##f(x) = 0## we have
##( x - \alpha_1 ) \ ... \ ... \ ( x - \alpha_r )( x^2 + \lambda_1 x + \mu_1 ) \ ... \ ... \ ( x^2 + \lambda_s x + \mu_s ) = 0##
As ##D## is a division algebra, one of the factors must be ##0##. ... ... "
My question is ... why does ##D## being a division algebra mean that one of the factors must be zero ...?
Help with questions 1 and 2 above will be appreciated .. ...
Peter
==============================================================================
In order for readers of the above post to appreciate the context of the post I am providing pages 1-2 of Bresar ... as follows ...


I need help with another aspect of the proof of Lemma 1.1 ... ...
Lemma 1.1 reads as follows:
My questions regarding Bresar's proof above are as follows:Question 1
In the above text from Bresar, in the proof of Lemma 1.1 we read the following:
"... ... As we know, ##f( \omega )## splits into linear and quadratic factors in ##\mathbb{R} [ \omega ]## ... ..."
My question is ... how exactly do we know that ##f( \omega )## splits into linear and quadratic factors in ##\mathbb{R} [ \omega ]## ... can someone please explain this fact ... ...
Question 2
In the above text from Bresar, in the proof of Lemma 1.1 we read the following:
" ... ... Since ##f(x) = 0## we have
##( x - \alpha_1 ) \ ... \ ... \ ( x - \alpha_r )( x^2 + \lambda_1 x + \mu_1 ) \ ... \ ... \ ( x^2 + \lambda_s x + \mu_s ) = 0##
As ##D## is a division algebra, one of the factors must be ##0##. ... ... "
My question is ... why does ##D## being a division algebra mean that one of the factors must be zero ...?
Help with questions 1 and 2 above will be appreciated .. ...
Peter
==============================================================================
In order for readers of the above post to appreciate the context of the post I am providing pages 1-2 of Bresar ... as follows ...


