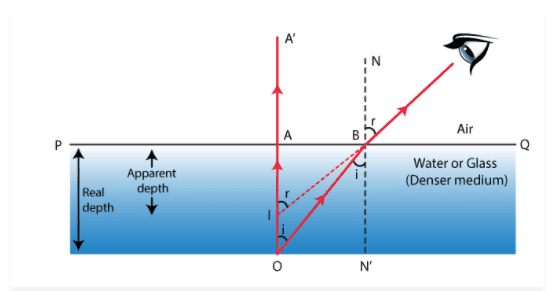
Mister T said:
It's where we think the coin is because that's where the image of the coin is formed. It may very well be that the image is not directly above the object, and it's a simplification made by textbook authors. I'd have to do a little research to find out.
Fair warning: this post is going to get long and has little to do with what the exercise is trying to get at.
The problem is that no clear image is formed at all.
One result of refraction through a flat surface is that the image is damaged. This is called "aberration". The upshot of this is that
the "apparent distance" to a object viewed through a flat refracting surface is not well defined.
One commonly finds textbooks talking about things like "spherical aberration" or "chromatic aberration".
Spherical aberration is, of course, the image damage that occurs when using a spherical refractor (or reflector) and looking at a portion of the image that is not exactly on the center axis. Rays from one point on the physical object will not all converge at a single point on the real image.
Chromatic aberration is, of course, the image damage that occurs when a refractor does not have the same refractive index for the relevant frequency range. Rays of different colors from a single point on the physical object will not all converge at a single point on the real image.
The aberration from looking through a flat refractive surface does not have a specific name that I know of. Like spherical aberration, it is a "geometrical aberration".
We can see what is going on by doing some ray tracing. First we will trace rays in a vertical plane, looking from one side as depicted in the OP. Next we will trace rays in a horizontal plane, looking down from above.
Say that we trace one ray that goes slightly above the line of sight and strikes the top edge of the pupil and another ray that goes slightly below the line of sight and strikes the bottom edge of the pupil. The eye attempts to focus and direct both of these rays on a single spot on the retina. The required distortion of the lens in the eye is a measurement of the divergence angle of these two rays and amounts to a triangulation -- a distance measurement.
It is hard to do the required calculations. Way harder than could be expected of the student solving this exercise. Way way harder.
Way harder than I am willing to attempt here. Suffice it to say that it is doable. One could carry out a calculation and figure out the distance to the intersection point where the two arriving rays would have originated if there were no water. All things being equal, this would be the position of the virtual image of the coin.
Now let us switch to the horizontal plane. We are looking from above. We do the ray tracing and trace rays going from the coin to the right edge of the pupil and to the left edge of the pupil. Again, the lens of the eye (we hope) causes both rays to converge on a single point on the retina. Again, we can can see that this amounts to a triangulation. Another distance measurement.
This time the required calculations are not hard. They are easy. Dead simple in fact.
Refraction through a horizontal surface causes zero deflection in the horizontal plane.
The triangulated horizontal distance to the virtual image is exactly equal to the actual horizontal distance to the coin.
The two distance measurements give two different results. We have aberration.
In practice, the head will be held upright. The two eyes will have a horizontal separation. The distance cue from binocular vision will be preferred to the ambiguous distance cue from lens tension.
Trust me on this, I can control both aspects of my vision somewhat independently. It is amusing to watch through one's eyes as the lens goes through autofocus and acquires an image at a different focal depth than binocular vision calls for. In normal operation, binocular vision and autofocus are strongly coordinated and binocular vision is highly constrained to face-relative horizontal. It takes practice to de-couple them. However, for some people (like my wife) the decoupling is involuntary and can manifest as strabismus or amblyopia, so one may wish to exercise due care before staring into a pegboard wall working to mis-train ones only set of eyes.We have seen above that distance triangulation in the horizontal plane is accurate. So we can return to the original problem. We have an assumed accurate horizontal distance estimate which we accepting as a given in order attempt a vertical distance estimation: How deep is the virtual image, given that its horizontal distance is as specified. The required calculation is simple and is what the exercise asks for.