Application of Newton's second law to a horizontal pulley

In summary, static friction is a variable reaction force with a maximum possible magnitude of μN. If the net external force on a box is less than μN, the static friction force will have the same magnitude but in the opposite direction. To make the net external force 0, an equal and opposite force of 20N needs to be applied. The minimum and maximum possible forces to make the system static are 14N and 26N respectively, as long as the net external force is less than μN.
  • #1
ElectroMaster88
3
3
Homework Statement
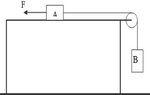
Body A, which has a mass of 3 kg, is on a horizontal surface and is connected by a massless and frictionless string and pulley to body B, which is suspended in the air and has a mass of 2 kg. The coefficient of friction (static and kinetic) between body A and the horizontal surface is 0.2.
A horizontal force F is applied to body A to the left.
A. Calculate what the value of the force F should be in order for the frictional force between body A and the surface to be zero.
B. In what range of values should the force F be in order for the system to be in a static state? There are answers:
A. 20N
B. 14N ≤ F ≤ 26N
Relevant Equations
x=x0+v0t+at^2/2
v=v0+at
f=μ*N
N=mg
Newton's Second Law
I don't understand how to solve these, and I don't understand how an horizontal force can affect the friction force if it's defined by μ*N, and the additional force affect neither of those. I also don't understand how there is a range of possible forces that F can be to make the system static, if only one option can make the horizontal net force 0.
I am clearly missing something and I don't understand what.
 

Attachments

  • problem.png
    problem.png
    8.1 KB · Views: 92
Physics news on Phys.org
  • #2
ElectroMaster88 said:
I am clearly missing something and I don't understand what.
Static friction is a variable reaction force. It is not a force with a fixed magnitude. The quantity ##\mu N## is the maximum possible force of static fiction. If the box is free from external forces, then the static friction acting on it is zero. If the net external force on the box is less than ##\mu N##, then the static friction force will have precisely the same magnitude as the new external force, but in the opposite direction.
 
  • Like
Likes berkeman and Lnewqban
  • #3
I think I understood:
Because the tension of the string is 20N, to make the net external force on box A 0 (which will make static friction of 0), I need to apply 20N of force in the opposite direction, which is F.
So that's why the answer is 20N.

And because μN is the maximum possible force of static friction (which is in this case 6N), at minimum I need to apply 14N of force to the opposite direction (20-6=14), and at maximum 26N, which will make the same scenario like in the 14N but with switched sides.
So as long as the net external force on the box is less than μN, the system will stay static.
Have I understood it right?
 
  • Like
Likes Lnewqban and PeroK

FAQ: Application of Newton's second law to a horizontal pulley

What is Newton's second law of motion?

Newton's second law of motion states that the acceleration of an object is directly proportional to the net force acting on it and inversely proportional to its mass. The law is commonly expressed with the formula: F = ma, where F is the net force, m is the mass, and a is the acceleration.

How do you apply Newton's second law to a horizontal pulley system?

To apply Newton's second law to a horizontal pulley system, you need to consider the forces acting on each mass separately. For each mass, write the equation F = ma, where the net force F is the difference between the tension in the string and any other forces acting on the mass (such as friction). Then, solve these equations simultaneously to find the acceleration and the tension in the string.

What role does tension play in a horizontal pulley system?

Tension is the force transmitted through the string or rope in a pulley system. In a horizontal pulley system, the tension force acts on both masses connected by the string. It is responsible for accelerating the masses and is a crucial component in applying Newton's second law to determine the system's behavior.

How does friction affect the application of Newton's second law in a horizontal pulley system?

Friction opposes the motion of the masses in a horizontal pulley system. When applying Newton's second law, you must include the frictional force in the net force calculation. The frictional force can be calculated using the coefficient of friction and the normal force. This force will reduce the net force acting on the masses, thereby affecting the acceleration.

Can you provide an example problem involving a horizontal pulley and Newton's second law?

Consider two masses, m1 and m2, connected by a string over a frictionless pulley. Mass m1 is on a horizontal table with friction coefficient μ, and mass m2 hangs off the side. For m1, the net force is T - μm1g = m1a, where T is the tension and g is the gravitational acceleration. For m2, the net force is m2g - T = m2a. Solving these equations simultaneously gives the acceleration a and the tension T in the string.

Similar threads

Newton's second law (My turtle named Newton being accelerated)
Replies
9
Views
2K
Newton's law problem: Pushing 2 stacked blocks on a horizontal table
Replies
13
Views
2K
Proving Newton's 3rd law from conservation of momentum
Replies
2
Views
368
Having a hard time learning Newton's 2nd law
Replies
5
Views
929
Friction and an object stopping
Replies
12
Views
2K
Static Friction Required to Keep the System from Moving (Two Boxes)
Replies
16
Views
2K
Relationship between horizontal force and friction in an exercise
Replies
7
Views
743
Unbalanced force to find altitude of airplane
Replies
4
Views
1K
Newton’s Law of Motions: tension forces in a pulley
Replies
4
Views
1K
Newton's laws — Two stacked blocks sliding on a frictionless floor
Replies
17
Views
2K

Hot Threads

Recent Insights

Back
Top