abdossamad2003
- 68
- 4
In Halliday's physics book, there is an example of the first law of thermodynamics that shows its application. The figure below explains this example:

Here is a question, if the element alone is chosen as the system, doubts arise in the first law, because in this system, Q<0 (because heat is transferred from the element to the water) and W=0 (because there is no external work on the system is not done) as a result the internal energy of the system (the element alone) should decrease while it does not because the temperature of the element is constant.!!!Part of the text:
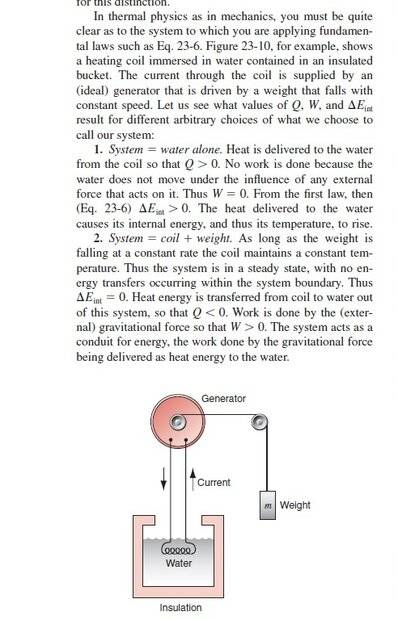
Here is a question, if the element alone is chosen as the system, doubts arise in the first law, because in this system, Q<0 (because heat is transferred from the element to the water) and W=0 (because there is no external work on the system is not done) as a result the internal energy of the system (the element alone) should decrease while it does not because the temperature of the element is constant.!!!Part of the text: