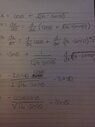Applications of differentiation

[Colin2]

I need help solving all three parts to this question, never seen a question regarding applications of differentiation that is this hard before!

View attachment 4094

All help is much appreciated.

 

[MarkFL]

We are given the function:

$$x=\cos(\theta)+\sqrt{16-\sin^2(\theta)}$$

This is the position function...how can we find the velocity so that we may equate it to zero and solve?

 

[Colin2]

View attachment 4096

I found the first derivative to obtain the velocity, but I'm stuck here.
I also don't know what to do with the value that is given for theta.

 

[MarkFL]

You are neglecting to apply the chain rule. You are given:

$$x=f(\theta)$$

And so we must have:

$$\d{x}{t}=\d{f}{\theta}\cdot\d{\theta}{t}$$

Now, you have correctly computed:

$$\d{f}{\theta}=-\sin(\theta)\left(1+\frac{\cos(\theta)}{\sqrt{16-\sin^2(\theta)}}\right)$$

We are given:

$$\theta=\frac{\pi}{2}t$$

So, what is $$\d{\theta}{t}$$?

 

[Colin2]

I kinda forgot that I posted this, anyone still willing to help me solve this just for the sake of solving it?
Any help is much appreciated!

 

[Sudharaka]

I kinda forgot that I posted this, anyone still willing to help me solve this just for the sake of solving it?
Any help is much appreciated!

Hi Colin,

Have you tried to follow what Mark has shown above. Did you find $\frac{d\theta}{dt}$?