Apply the Legendre Transformation to the Entropy S as a function of E

[GravityX]

Homework Statement

Apply Legendre Transformation to Entropy

Relevant Equations

$g(m)=f(x(m))-m*x(m)$ and $x(m)=(f')^-1(m)$

Hi,

Unfortunately I am not getting anywhere with task three, I don't know exactly what to show

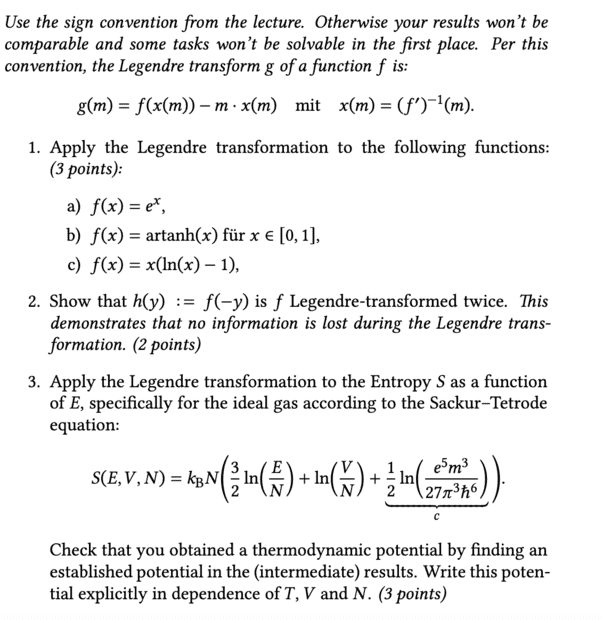
Shall I now show that from $S(T,V,N)$ using Legendre I then get $S(E,V,N)$ and thus obtain the Sackur-Tetrode equation?

 

[vanhees71]

You should rather think in terms of differentials. The "natural independent parameters" for $S$ are $(E,V,N)$. Now you want to get another potential with the "natural independent parameters" $(T,V,N)$. So first write down the differential $\mathrm{d} S$ in terms of $(E,V,N)$ and then think about, how to Legendre transform to a new potential with the other set of independent parameters.

 

[GravityX]

Thanks vanhees71 for your help

I have now represented $ds$ as follows

$$ds=\frac{\partial S}{\partial E}dE+\frac{\partial S}{\partial V}dV+\frac{\partial S}{\partial N}dN$$

$$ds=\frac{1}{T}dE+\frac{P}{T}dV-\frac{\mu }{T}dN$$

Now I would just have to get rid of the $dE$ or rather I would have to express $dE$ with the help of $dT$, $dV$, $dN$, right?

 

[vanhees71]

Write it in the form of $\mathrm{d} E=...$ then find a new potential, $F$ ("free energy"), such that instead of a differnetial with $\mathrm{d} s$, $\mathrm{d} V$ and $\mathrm{d}N$ you get one with $\mathrm{d} T$, $\mathrm{d}V$ and $\mathrm{d}V$. Note that
$$\mathrm{d}(Ts)=s \mathrm{d} T + T \mathrm{d} s!$$

 

[GravityX]

Thanks for your help vanhees71 I think I got it now