- #1
schniefen
- 178
- 4
- Homework Statement
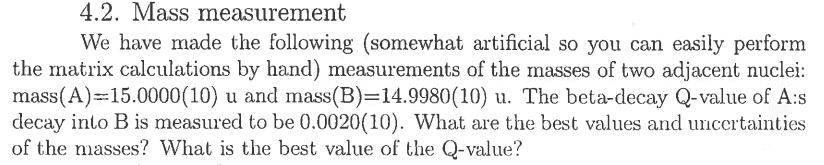
- See attached image.
- Relevant Equations
- Q-value: The difference in mass between the mother atom and the daughter atom.
Consider the problem in the attached image. The difference between A and B is 0.0020(20). How does one use the least squares method, particularly in matrix form, to find the best value of the masses of A and B respectively, as well as the Q-value? Aren't more measurements needed for the masses and the Q-value?