How to Calculate Surface Integral Using Stokes' Theorem?

I
Thread starter WMDhamnekar
Start date Nov 30, 2022
Tags

Line integral Stokes Surface integral Theorem

In summary, Stokes' theorem is a fundamental theorem in vector calculus that relates the surface integral of a vector field to the line integral of its curl over the boundary of the surface. It is used when evaluating integrals in three-dimensional space, particularly when the region of integration is a closed surface. Its significance lies in its ability to provide a powerful tool for calculating surface integrals, making it useful in many areas of physics and engineering. In order to apply it, the surface must be closed and have a smooth and simple boundary curve, and the vector field must be differentiable in the region of integration. Stokes' theorem can also be generalized to higher dimensions, relating the integral over an n-dimensional manifold to the (n-1)-dimensional

Nov 30, 2022

WMDhamnekar

MHB

TL;DR Summary: Stokes’ theorem translates between the flux integral of surface S ##\displaystyle\iint\limits_{\Sigma} f \cdot d\sigma ## to ## \displaystyle\int\limits_C f\cdot dr## a line integral around the boundary of S. Therefore, the theorem allows us to compute surface integrals or line integrals that would ordinarily be quite difficult by translating the line integral into a surface integral or vice versa.
Calculating a surface integral

Calculate surface integral ## \displaystyle\iint\limits_S curl F \cdot dS ## where S is the surface, oriented outward in below given figure and F = [ z,2xy,x+y].

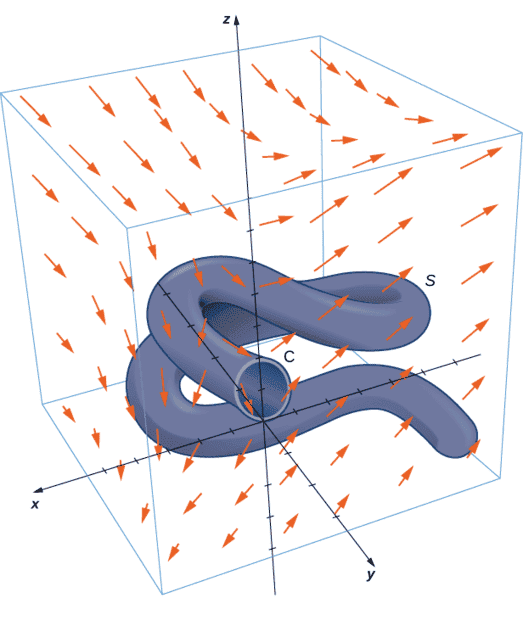
How can we answer this question?

Physics news on Phys.org

Nov 30, 2022

pasmith

Science Advisor

Homework Helper

You would do that as a line integral over [itex]C[/itex]; I assume the question tells you what [itex]C[/itex] is?

What is Stokes' theorem?

Stokes' theorem is a fundamental theorem in vector calculus that relates the surface integral of a vector field over a closed surface to the line integral of the same vector field along the boundary of the surface.

What is the significance of Stokes' theorem?

Stokes' theorem is significant because it allows for the calculation of a surface integral, which can be a difficult and time-consuming task, by converting it into a simpler line integral. This theorem is also used in many areas of physics and engineering, such as fluid mechanics and electromagnetism.

What are the conditions for applying Stokes' theorem?

In order to apply Stokes' theorem, the surface must be closed, meaning it has a boundary, and the vector field must be differentiable in the region enclosed by the surface.

How is Stokes' theorem related to Green's theorem?

Stokes' theorem is a generalization of Green's theorem, which relates a line integral around a simple closed curve to a double integral over the region enclosed by the curve. Stokes' theorem extends this concept to surfaces in three dimensions.

Can Stokes' theorem be applied to any surface and vector field?

Stokes' theorem can be applied to any smooth surface and any differentiable vector field in the region enclosed by the surface. However, for some surfaces and vector fields, the calculations may be more complex and difficult to solve.