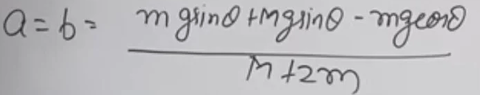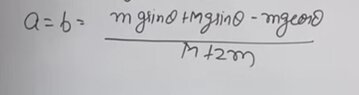Differentiate it
- 63
- 2
- Homework Statement
- There is no friction, and the pulley is massless. What is the acceleration of mass M?
- Relevant Equations
- F = ma
Is my answer correct? And if not, why?
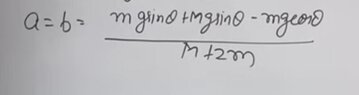
 .
.