- #1
raisins
- 3
- 1
Hi all,
I'm reading through Chapter 22 of Ashcroft and Mermin and am having difficulty deriving an equation. Could someone please show me (or outline the steps) how Ashcroft and Mermin convert the quadratic term in Eqn. (22.6) to Eqn. (22. 9)? (pictures attached).
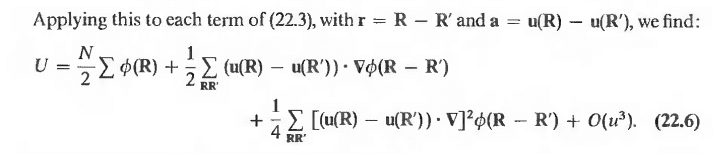

Thanks in advance :)
I'm reading through Chapter 22 of Ashcroft and Mermin and am having difficulty deriving an equation. Could someone please show me (or outline the steps) how Ashcroft and Mermin convert the quadratic term in Eqn. (22.6) to Eqn. (22. 9)? (pictures attached).
Thanks in advance :)