- #1
Jdtbtb_sp
- 4
- 0
May I know how to calculate this question without a calculator? The final answer of this question is 3 but I really have no idea how to work on it to get the final answer.
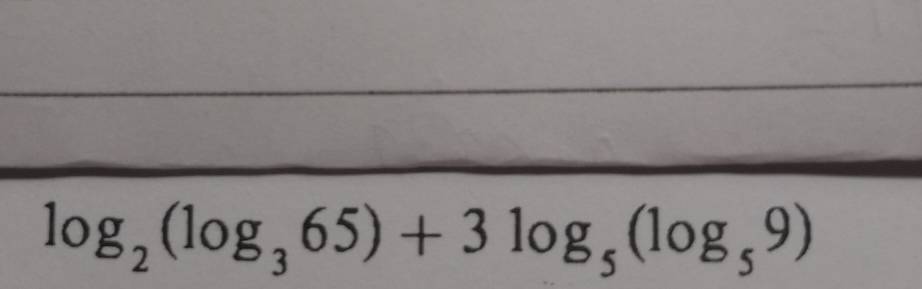
Last edited:
(ask) Calculating Logarithmic Question
- MHB
- Thread starter Jdtbtb_sp
- Start date
-
- Tags
- Logarithmic
In summary, the conversation discusses the difficulty of calculating a question without a calculator. The final answer is given as 3, but the individual is unsure of how to reach this answer without a calculator. The use of the changing base formula and conversion to the same base is also mentioned, but it is concluded that a calculator is necessary. The answer given in the book and the calculator's result are also slightly different due to rounding off. The individual thanks the other person for their response and acknowledges the need to use a calculator for similar questions in the future.
- #2
Jdtbtb_sp
- 4
- 0
Is it something to do with the changing base formula? I have tried using the changing base formula, hoping through this I could simplify it but it went even complicated. Then I was stuck, don't know how to solve it...Jdtbtb_sp said:
Last edited:
- #3
skeeter
- 1,103
- 1
Wolfram Alpha & my calculator do not get an evaluation that equals 3 ...
log_2(log_3(65)) + 3*log_5(log_5(9)) - Wolfram|Alpha
log_2(log_3(65)) + 3*log_5(log_5(9)) - Wolfram|Alpha
- #4
HOI
- 921
- 2
Even if you change all the logarithms to the same base there is not going to be any simple way to calculate that "log(log)" without a calculator. That's why calculators were invented!
- #5
Jdtbtb_sp
- 4
- 0
skeeter said:
The answer given in my book is 3. I used a calculator and it also gave me 2.506 as well, perhaps it is because of rounding off.
- #6
Jdtbtb_sp
- 4
- 0
Country Boy said:
Thank you for replying. Next time I should use a calculator for such a "log(log)" question. Thank you for spending time to answer my post.
1. What is a logarithm?
A logarithm is the inverse operation of exponentiation. It is a mathematical function that helps us solve for the exponent in an exponential equation. For example, in the equation 2^x = 8, the logarithm (base 2) of 8 is 3, since 2^3 = 8.
2. How do you calculate logarithms?
To calculate a logarithm, you need to know the base and the number you are taking the logarithm of. For example, if you want to calculate log base 10 of 100, you would write it as log10100. This is read as "log base 10 of 100." The answer would be 2, since 10^2 = 100.
3. What is the difference between natural logarithms and common logarithms?
Natural logarithms, written as ln(x), use the base e (Euler's number) and are often used in calculus and other advanced mathematical concepts. Common logarithms, written as log(x), use the base 10 and are more commonly used in everyday calculations.
4. What are some common uses for logarithms?
Logarithms are used in a variety of fields, including finance, science, and engineering. They are commonly used to measure the intensity of earthquakes (Richter scale), the loudness of sounds (decibels), and the acidity of substances (pH scale). They are also used in compound interest calculations and to solve exponential growth and decay problems.
5. Are there any rules or properties of logarithms?
Yes, there are several rules and properties of logarithms that can be used to simplify calculations. Some of the most common ones include the product rule, quotient rule, and power rule. These rules can help us solve complex logarithmic equations more easily.
Similar threads
-
General Math
-
General Math
-
General Math
-
General Math
-
Precalculus Mathematics Homework Help
-
General Math
-
General Math
-
General Math
-
Precalculus Mathematics Homework Help