Astrolabe Roadstead tides - Sinusoidal Functions

[Evangeline101]

Homework Statement

Homework Equations

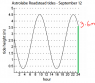
3. The Attempt at a Solution a) The height of the high tide is 4.5 m

b) The height of the low tide is 0.25 m

c)

Period = 12.5 hours k= 360/12.5 = 28.8
amplitude = 2.125 m
vertical shift = 2.375 m
phase shift = it doesn't look like there is any phase shift (correct if i am wrong please)

So the equation is:

-2.125 sin(28.8x) + 2.375

Is my equation correct?

d)

Determine height of tide at 5:00 pm:

y= -2.125 sin (28.8x) + 2.375

y= -2.125 sin (28.8(17)) + 2.375

y = -2.125 sin(489.6) +2.375

y = 0.737 m

The height of the tide at 5:00 pm is 0.74 m.

When would be a better time for the fisherman to come in?

If the fisherman comes into shore at 5:00 p.m. there will be a low tide with a height of 0.74 m. Since sandbars occur at a low tide (I am assuming) it would be best to come in when the tides are high to avoid getting stuck.

For example:
The fisherman could come in a few hours after 5:00 pm, between 8:00 pm to 11:00 pm, to catch the high tide. Please look over my answers and point out any mistakes :)

Thanks.

 

[tech99]

The low tide looks about 0.3m to me but otherwise your answers look OK as far as I can see.
I presume you are studying maths, not seamanship, but as a matter of interest, there is a "rule of twelfths", which give quite good answers without using sines at the same time as steering and keeping lookout! The overall tidal rise is 4.25 m, so in the first hour it rises about 1/12 x 4.25 = 0.35m, giving a total height of 0.25+0.35 = 0.6m. Not bad.
The full rule is as follows:
Hour 1, rise is 1/12
Hour 2, rise is 2/12
Hour 3, rise is 3/12
Hour 4, rise is 3/12
Hour 5, rise is 2/12
Hour 6, rise is 1/12..

 

[haruspex]

Period = 12.5 hours

12.4 is closer, and I agree with tech99 that the minimum looks more like 0.3 than 0.25.

it doesn't look like there is any phase shift (correct if i am wrong please)

What height does your equation give for midnight? What does the graph show?

 

[Evangeline101]

The low tide looks about 0.3m to me but otherwise your answers look OK as far as I can see.

12.4 is closer, and I agree with tech99 that the minimum looks more like 0.3 than 0.25.

Ok so by changing the height of the low tide to 0.3 m and the period to 12.4, the new equation so far would be:

y= -2.1 sin (29x) + 2.4

What height does your equation give for midnight? What does the graph show?

At midnight my equation gives a height of 3.25 m, which is obviously not what the graph shows.

So here is the new equation:

-2.1 sin (29(x-0.4)) +2.4

Using this equation the height at midnight is 3.6 m, which is what the graph shows.

Does this look right?

 

[haruspex]

Ok so by changing the height of the low tide to 0.3 m and the period to 12.4, the new equation so far would be:

y= -2.1 sin (29x) + 2.4
At midnight my equation gives a height of 3.25 m, which is obviously not what the graph shows.

So here is the new equation:

-2.1 sin (29(x-0.4)) +2.4

Using this equation the height at midnight is 3.6 m, which is what the graph shows.

Does this look right?

Not sure how you get the -.4. Looks too much subtraction to me. I plugged in y(0)=2.6 and got x-0.2. The height at midnight is not 3.6m, more like 3.1m. I think you did not follow the right line of dots up.

 

[Evangeline101]

The height at midnight is not 3.6m, more like 3.1m. I think you did not follow the right line of dots up.

midnight = 12 am = 24 hours

At 24 hours, the height of the tide is about 3.6 m

(the green line is the line of dots i followed)

That's how i got 3.6 m.

 

[Evangeline101]

Accidentally posted the same response twice

 

[haruspex]

midnight = 12 am = 24 hours

How true
You are probably close enough. To get a better fit would require a proper regression analysis. This is not difficult with a sine curve once you have the period reasonably accurate.

 

[Evangeline101]

Not sure how you get the -.4. Looks too much subtraction to me.

I got 0.4 as the phase shift by looking at the graph and estimating, i took the midpoint (2.4) and looked at how far the curve was from the y-axis (if that makes any sense). I would say that the phase shift is 0.4 hours (24 minutes) to the right??

 

[haruspex]

I got 0.4 as the phase shift by looking at the graph and estimating, i took the midpoint (2.4) and looked at how far the curve was from the y-axis (if that makes any sense). I would say that the phase shift is 0.4 hours (24 minutes) to the right??

I understand that makes it work well at the 24 mark, but it's a bit off at the 0 mark. As I wrote, you are probably close enough.

 

[Evangeline101]

I understand that makes it work well at the 24 mark, but it's a bit off at the 0 mark. As I wrote, you are probably close enough.

Your right, 0.4 is a bit off at 0 (2.8) , but 0.2 works well at 0 and is a bit off at the 24 mark (3.4)

I know you said I'm probably close enough, but which do you think is better to use? .

 

[haruspex]

Your right, 0.4 is a bit off at 0 (2.8) , but 0.2 works well at 0 and is a bit off at the 24 mark (3.4)

I know you said I'm probably close enough, but which do you think is better to use? .

For the last part of the question, you need the accuracy to be in the last six hours, so use 0.4.

 

[Evangeline101]

For the last part of the question, you need the accuracy to be in the last six hours, so use 0.4.

Ok makes sense.

One more question, when I am stating the amplitude, should I say that the amplitude is -2.1 m or the amplitude is 2.1 m?

 

[haruspex]

Ok makes sense.

One more question, when I am stating the amplitude, should I say that the amplitude is -2.1 m or the amplitude is 2.1 m?

An amplitude is a magnitude, like a speed, so is never negative.

 

[Evangeline101]

An amplitude is a magnitude, like a speed, so is never negative.

Ok, thanks for all the help!