Average value of components of angular momentum for a wave packet

[Nelsonc]

Homework Statement

Given a wave packet as shown (see below), find the mean value of angular momentum components L_x, L_y, L_z with regard to point (a,0,-b) where a and b (the impact parameter) are nonzero

Relevant Equations

\frac{1}{\pi^{3/4} \sqrt{\sigma_x\sigma_y\sigma_z}}e^{-(x^2/2{\sigma_x}^2+y^2/2{\sigma_y}^2+z^2/2{\sigma_z}^2)}e^{i(p_0/\hbar)x}

I have typed up the main problem in latex (see photo below)

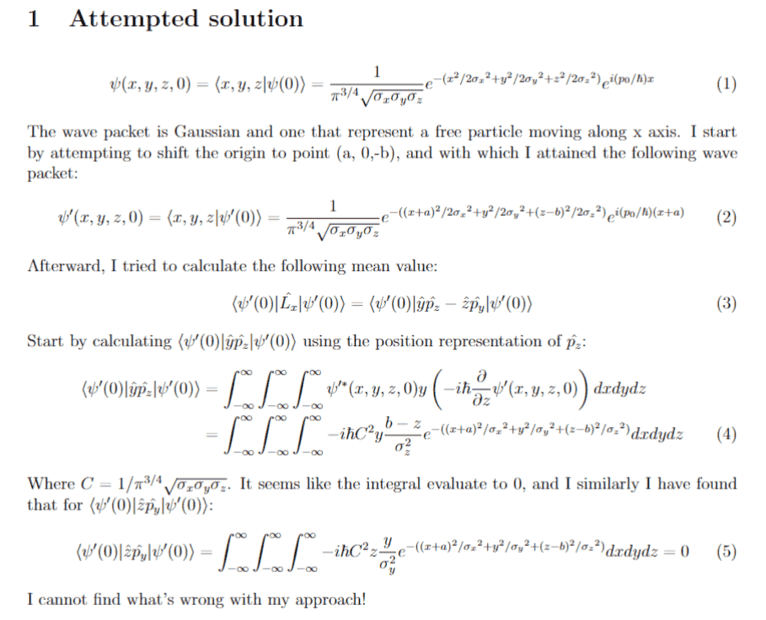
It seems all such integrals evaluates to 0, but that is apparantly unreasonable for in classical mechanics such a free particle is with nonzero angular momentum with respect to y axis.

 

[TSny]

It seems all such integrals evaluates to 0, but that is apparantly unreasonable for in classical mechanics such a free particle is with nonzero angular momentum with respect to y axis.

You didn't show your work for the y and z components of angular momentum. You shouldn't get zero for the y component.

 

[Nelsonc]

Thanks for the reminder, but I have already done so and it turns out they all goes to 0, so there must be something awry with my method. In particular, there seems always to be one spacial part of the integrand to be antisymmetric so that the whole integral goes to 0 (please refer to the image attached). Moreover, I know that classically a free particle moving in such fashion would have y angular momentum component being $bp_0$

 

[TSny]

In deriving equation (7), check your result for $\large \frac{\partial \psi'}{\partial x}$. Did you use the product rule when taking the derivative of the product of the Gaussian function and the function $e^{i(p_0/\hbar)x}$?

 

[Nelsonc]

I see, thanks so much for catching that error! Now the calculation generates $bp_0$ as a result.