Axial field from a pointed tip inside cylinder with flows

[Madoro]

Homework Statement

Hello, I have an experimental problem that I don´t know how to express theoretically.
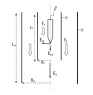
It consists on a tip at high voltage inside an earthed cylinder, where a discharge is produced. The discharge is radial, since is the shortest distance to the wall, and produces ions, which are dragged out by an air flow f₁, generating an electrical field in the axial direction E_x1 (fig. discharge.png).
There is an extra air flow f₂ that joins f₁ into a surrounding earthed cylinder, and then the field by the ions changes its value, E_x, since the flow is bigger and is further away from the discharge.

I have no clue neither how to calculate E_x1 nor E_x2. The attempt at a solution

I have tried to solve it supposing the discharge as a charged ring, similarly to how is done in this thread:
https://www.physicsforums.com/showthread.php?t=497126&highlight=radial+field+flow
Therefore, the electrical field at the output of the inner cylinder would be:
$E_{x1}=\frac{q}{4\pi \epsilon_0}\frac{L_i}{(L_i^2+R_i^2)^{3/2}}$

and therefore in the outer:
$E_x=E_{x1}+\frac{q}{4\pi \epsilon_0}\frac{L_o}{(L_o^2+R_o^2)^{3/2}}$

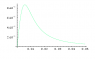
obtaining the solution shown in Ex.png.

First of all, is a good approximation the rings to the solution?
and secondly, why the axial field does this increase in the curve? should´t it be constantly decreasing?

Any help would be really appreciated, since I’m stuck here for long time now.
Thanks in advance.

 

[Madoro]

Second attempt:

I divide the problem in two parts, first inside the inner cylinder and then in the bigger one. I'll begin in the inner one where discharge is started
I suppose a radial discharge from the tip to the walls:
$E_r=\frac{U}{r Log(R_i/R_{tip})}$
being $U$ the applied voltage and $R_{tip}$ the radius of the needle's tip.

The governing equations (all in cylindrical coordinates) are the Poisson equation for the field inside the inner cylinder:
$\frac{1}{r}\frac{\partial (r E_r)}{\partial r}+\frac{\partial E_z}{\partial z}=\frac{\rho}{\epsilon_0}$ (1)

and the convection-diffussion equation for the charge density:
$\frac{\partial \rho}{\partial t}=D_i \nabla^2 \rho + \nabla \cdot (u \rho)=0$ (2)

where $D_i$ is the diffusion coefficient and is so small that this term can be neglected. Velocities in axial and radial directions are:
$u_r=Z_i E_r$
$u_z=u_g+Z_i E_z$
with $u_g$ the $f_1$ flow velocity.

Therefore eq. (2):
$\frac{1}{r}\frac{\partial (r Z_i E_r \rho)}{\partial r}+\frac{\partial ((u_g+ Z_i E_z) \rho))}{\partial z}=0$ (2b)

Solving eq. (1) and (2b):

$\frac{\partial E_z}{\partial z}=\frac{\rho}{\epsilon_0}$ (3)

$Z_i E_r \frac{\partial \rho}{\partial r}+(u_g+ Z_i E_z) \frac{\partial \rho}{\partial z} + \rho Z_i \frac{\partial E_z}{\partial z}=0$ (4)

Substituting eq. (3) into eq.(4):
$Z_i E_r \frac{\partial \rho}{\partial r}+(u_g+ Z_i E_z) \frac{\partial \rho}{\partial z} + \frac{\rho^2 Z_i}{\epsilon_0}=0$ (4b)


Therefore I have a system of 2 equations (3 & 4b) with 2 unknowns ($E_z$ and $\rho(z,r)$), but I'm totally stuck on how to solve it.

Please, correct me if my approach of the problem is not correct or there is any mistake in the resolution. I could use some software to solve the system of equations, but I'm not sure if they are resoluble.

Thanks in advance.