B.1.2.3 Find the equilibrium solution and....

[karush]

$\tiny{b.1.2.3}$

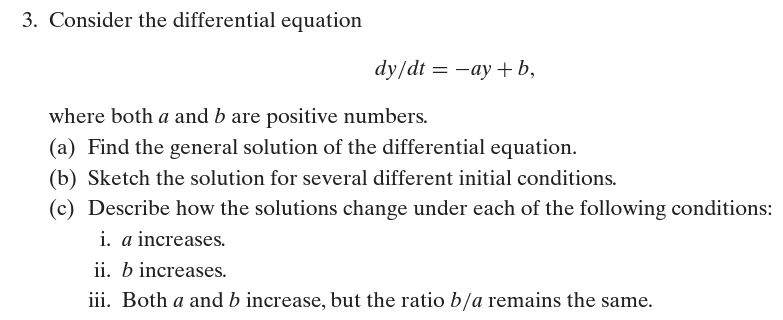
Consider the differential equation
$\displaystyle \dfrac{dy}{dt}=ay-b$
Find the equilibrium solution $y_e$ rewrite as
$y'=ay-b=0$
then
$ay-b=0\implies y_e=\dfrac{b}{a}$
(b) Let $Y(t)=y-y_e$;
thus $Y(t)$ is the deviation from the equilibrium solution.
the differential equation satisfied by $Y(t)$.

so far but ?here is the book answer

 

[HOI]

$\tiny{b.1.2.3}$
View attachment 11141
Consider the differential equation
$\displaystyle \dfrac{dy}{dt}=ay-b$
Find the equilibrium solution $y_e$ rewrite as
$y'=ay-b=0$
then
$ay-b=0\implies y_e=\dfrac{b}{a}$
(b) Let $Y(t)=y-y_e$;
thus $Y(t)$ is the deviation from the equilibrium solution.
the differential equation satisfied by $Y(t)$.

so far but ?

If Y= y-y_e the y= Y+ y_e.
Y'= y'= ay- b= a(Y+ y_e)= a(Y+ b/a)= aY+ b.
Y also satisfies the equation Y'= aY+ b.

With y'= dy/dx= ay+ b, dy/(ay+b)= dx.
To integrate the left, let u= ay+ b so that du= ady. dy= (1/a)du and the equation becomes
(1/a) du/u= dx
(1/a) ln(u)= x+ C
ln(u)= ax+ aC
u= e^{ax+ aC}= e^{aC}e^{ax}= C'e^{ax} where C'= e^{aC}
ay+ b= C'e^{ax}
ay= C'e^{ax}- b
y= (C'/a)e^{ax}- b/a= C''e^{ax}- b/a where C''= C'/a.

If a increases, the equilbrium, b/a, decreases but e^{ax} increases faster so y increases faster.
If a decreases. the equilibrium, b/a, increases but e^{ax} decreases slow so y increases slower.

here is the book answer

View attachment 11140

 

[karush]

mahalo helps a lot