Ballentine Equation 5.13 on conservation of momentum

[EE18]

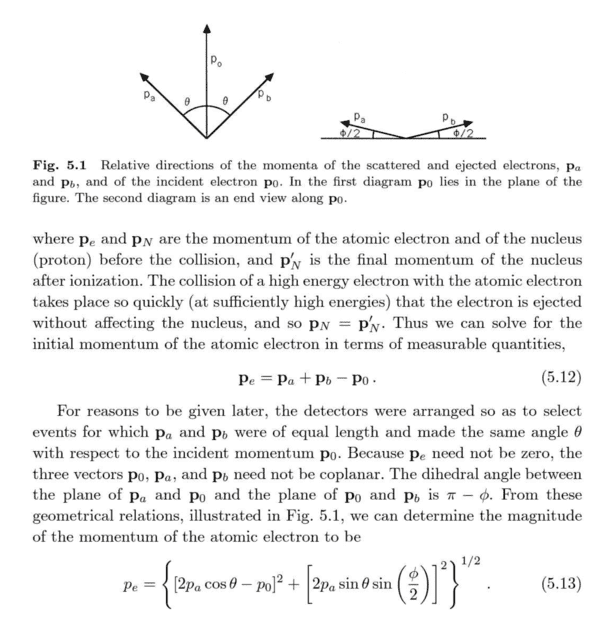
In Chapter 5.3, Ballentine uses geometrical arguments to obtain the initial magnitude of a hydrogen atom's bound electron momentum. How does equation (5.13) obtain? I tried to naively compute
$$p_e^2 \equiv \textbf{p}_e\cdot \textbf{p}_e = p_a^2+p_b^2+p_o^2 + 2\textbf{p}_a\cdot \textbf{p}_b - 2\textbf{p}_a\cdot \textbf{p}_0 - 2\textbf{p}_0\cdot \textbf{p}_b $$ $$= p_a^2+p_b^2+p_o^2 + 2p_ap_b\cos(\pi - \phi) - 2p_ap_0\cos \theta - 2p_bp_0\cos \theta$$
but then could not go any further. Am I misunderstanding the geometrical relationships of the vectors in Figure 5.1?

 

[TSny]

$$p_e^2 \equiv \textbf{p}_e\cdot \textbf{p}_e = p_a^2+p_b^2+p_o^2 + 2\textbf{p}_a\cdot \textbf{p}_b - 2\textbf{p}_a\cdot \textbf{p}_0 - 2\textbf{p}_0\cdot \textbf{p}_b $$ $$= p_a^2+p_b^2+p_o^2 + 2p_ap_b\cos(\pi - \phi) - 2p_ap_0\cos \theta - 2p_bp_0\cos \theta$$
Am I misunderstanding the geometrical relationships of the vectors in Figure 5.1?

The angle between $\mathbf{P}_a$ and $\mathbf{P}_b$ is not $\pi - \phi$.

$\mathbf{P}_a$ lies in the yellow plane that makes angle $\phi/2$ to the horizontal gray plane. You might try finding expressions for the x, y, and z components of $\mathbf{P}_a$ (shown in blue).

 

[EE18]

The angle between $\mathbf{P}_a$ and $\mathbf{P}_b$ is not $\pi - \phi$.

$\mathbf{P}_a$ lies in the yellow plane that makes angle $\phi/2$ to the horizontal gray plane. You might try finding expressions for the x, y, and z components of $\mathbf{P}_a$ (shown in blue).

View attachment 324201

Thank you so much for that diagram, it helps me tremendously.

It seems like I have, by symmetry, that $\textbf{p}_a \cdot \textbf{p}_b = p_{ax}^2 -p_{ay}^2 + p_{az}^2$. It then remains to find these components in terms of the given angles and $p_a$. Now clearly $p_{az} = \tan(\phi/2)p_{ay}$, $p_{az} = p_a \cos \theta$, and $p_a^2 = p_{ax}^2 +p_{ay}^2 + p_{az}^2$ so that at least in theory I have three equations with which I can substitute away $p_{ax}^2 -p_{ay}^2 + p_{az}^2$ in the above in terms of the angles and $p_a$. However it seems very ugly -- is there a cleaner way to do it or is it necessarily ugly?

 

[TSny]

Now clearly $p_{az} = \tan(\phi/2)p_{ay}$, $p_{az} = p_a \cos \theta$

I think you meant the second equation to represent $p_{ax}$.

Consider writing $\mathbf{p}_a$ in unit vector notation $$\mathbf{p}_a =p_{ax} \mathbf{i} +p_{ay} \mathbf{j} +p_{az} \mathbf{k}$$ Each of the components can be expressed in terms of the magnitude $p_a$ and the angles $\theta$ and $\phi/2$. For example, you know $p_{ax} = p_a \cos \theta$.

Do the same for $\mathbf{p}_b$.

For $\mathbf{p}_o$ we have simply $\mathbf{p}_o = p_0 \mathbf{i}$. Then use equation (5.12) to find the component expression for $\mathbf{p}_e$.