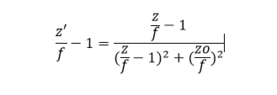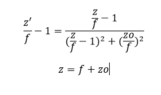tanhanhbi
- 9
- 3
- Homework Statement
- Determine the optical distance d between the lenses such that the distance z′ to the waist of the final beam is as large as possible.
- Relevant Equations
- Gaussian beam
Basically this is the Exercise In Fundamentals of Photonics book.
We also need to use these two equation (1) and (2)
As we all know, in order to make the z' as far as possible, we must place 2 lens with this distance
I already figure that thank to the initial condition of the first lens position
So that the z1 must equal to f1
For the z2, I tried to replace zo in (2) onto (1), then take the derivative of z' with respect to z2. My initial though that let the derivative of z' equal to 0, we can find the maximal of z'. But actually after finish the derivative thing, z' do not have maximal and I already always infinity ?
So I am not sure what wrong with my thinking. If someone could give me a clue, I really appreciate it.