plmustard
- 2
- 0
- TL;DR Summary
- Assuming entangled photons created with a horizontal/vertical basis, does demonstrating Malus' law in a Bell Test require one of the polarizing filters to be set at either the horizontal or vertical basis?
Qutools makes quantum physics kits for educational purposes. Its quED kit is designed to help students learn about entanglement by performing Bell tests. In the manual section 5.1 it describes "the simplest test to verify entanglement of photon pairs."
My question is if the entangled photons have a horizontal/vertical basis as described (where 0 degrees corresponds to horizontal polarization), but the alpha polarizing filter is fixed to 30 degrees instead of 0 degrees as described, would the rotation of the beta filter still result in correlations between the two filter settings consistent with Malus' law such that sin^2 (beta - alpha)? In other words if alpha was at 30 degrees and beta at -30 degrees (or 330 degrees), and the system is set to a horizontal/vertical basis, would the photons be different 75% of the time because sin^2 (-30 - 30) = sin^2 (-60) = 0.75? Or would that calculation only apply if alpha (or beta) were fixed at 0 degrees because of the photon horizontal/vertical basis being at 0/90 degrees? Thank you for any explanation.
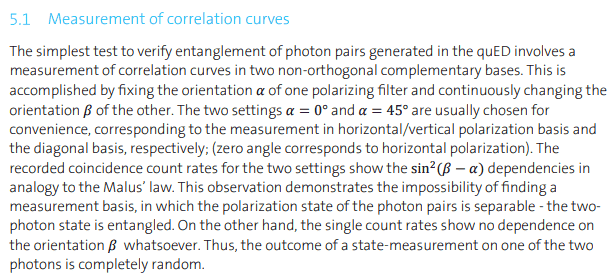
My question is if the entangled photons have a horizontal/vertical basis as described (where 0 degrees corresponds to horizontal polarization), but the alpha polarizing filter is fixed to 30 degrees instead of 0 degrees as described, would the rotation of the beta filter still result in correlations between the two filter settings consistent with Malus' law such that sin^2 (beta - alpha)? In other words if alpha was at 30 degrees and beta at -30 degrees (or 330 degrees), and the system is set to a horizontal/vertical basis, would the photons be different 75% of the time because sin^2 (-30 - 30) = sin^2 (-60) = 0.75? Or would that calculation only apply if alpha (or beta) were fixed at 0 degrees because of the photon horizontal/vertical basis being at 0/90 degrees? Thank you for any explanation.