Block tied to a fixed string on an accelerating wedge

[Null_Void]

Homework Statement

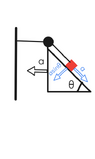
We have a block on a wedge, connected to a string which is fixed on a vertical wall to the left of the wedge.The string is horizontal and taut.
The wedge has an acceleration a towards the right. Mass of block is m.

Find the acceleration of the block
i) Relative to the ground
ii) Relative to the block

There is no friction anywhere.

Relevant Equations

Acceleration a along the incline

T - mg = ma

Acceleration along the normal to the incline

mgcosø - N = masinø

So I basically identified two constraints,

1) The string cannot be slack, therefore the acceleration of block down the incline should be equal to the acceleration of the wedge.

2) The block must always stay in contact with the wedge. Hence, the acceleration of the block normal to incline must be equal to the component of the wedge's acceleration along that directi
on.

The answer is given as option A and B which is absolutely not what I'm getting.
So what am I missing here ?
Is there anything wrong with my inference?


Here is a picture of the question,

 

[Orodruin]

(A) is essentially your first constraint …

 

[haruspex]

The answer is given as option A and B

But it says only one is correct?