Bob's question at Yahoo Answers regarding equilibrum points of ODE

[MarkFL]

Here is the question:

Differential Equations Harvesting of Fish?

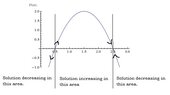
Suppose the growth of the population P=P(t) of a certain fish species in a lake can be modeled by the differential equation dP/dt=kP(1-P/N), where k is the growth rate factor and N is the carrying capacity of the lake. Now, suppose that fishing removes a certain number H of fish per season from the population, then the equation can be modified as dP/dt/kP(1-P/N)-H. The positive constant H is called the harvesting factor.

1. Find the equilibrium points H< kN/4 and show that for any sufficient large initial population Po=P(0), the population of the fish will eventually stabilize at certain level, and this equilibrium level, will decrease gradually to N/2 as the harvesting factor H increases toward kN/4

2. Find the equilibrium points and show that as soon as H>kN/4, regardless how large the initial population Po, all the fish in the lake will be wiped out. Note: You need to show that the rape dP/dt is less than some fixed negative number, so that given enough time, the population will have to decrease to 0.

If you can only help me one of these, i would still greatly appreciate the help!

I have posted a link there to this thread so the OP can view my work.

 

[MarkFL]

Hello Bob,

We are given the ODE:

\(\displaystyle \frac{dP}{dt}=kP\left(1-\frac{P}{N} \right)-H\)

To find the equilibrium points, we want to look at:

\(\displaystyle \frac{dP}{dt}=0=kP\left(1-\frac{P}{N} \right)-H\)

This is, we want to look at the condition(s) necessary for the solution to have no change, or a slope of zero. So, we have:

\(\displaystyle kP\left(1-\frac{P}{N} \right)-H=0\)

Let's arrange this in standard quadratic form in $P$:

\(\displaystyle kP^2-kNP+HN=0\)

Application of the quadratic formula yields:

\(\displaystyle P=\frac{kN\pm\sqrt{k^2N^2-4kHN}}{2k}\)

Now, in order for these roots to be real, we require the discriminant to be non-negative:

\(\displaystyle k^2N^2-4kHN\ge0\)

Assuming $0<k,N$, we have:

\(\displaystyle kN-4H\ge0\)

which we may arrange as:

\(\displaystyle H\le\frac{kN}{4}\)

1.) We are given:

\(\displaystyle H<\frac{kN}{4}\)

And so we know we are assured of two real AND distinct equilibrium points, given by:

\(\displaystyle P=\frac{kN\pm\sqrt{kN(kN-4H)}}{2k}\)

We can also see that both roots are positive, since:

\(\displaystyle kN=\sqrt{k^2N^2}>\sqrt{kN(kN-4H)}=\sqrt{k^2N^2-4kHN}\)

Now, If we go back to:

\(\displaystyle kP\left(1-\frac{P}{N} \right)-H=0\)

We see that we have a parabolic function, opening downward. This tells us the slope of the solution is negative below the smaller root, positive in between the two roots, and negative above the larger root.

So, what we may conclude from this is that for any initial value $P(0)$ of the fish population that is less than the smaller equilibrium point the population will decrease, approaching zero as time passes, and the fish will become extinct. If the initial population is equal to the smaller equilibrium point, then the population will remain unchanged over time. And for any initial population greater than the smaller equilibrium point all solutions will approach the larger equilibrium point. Look at the following diagram:

View attachment 2030

Ignore the actual numbers on the axes, and just look at the behavior we find for the solution to the IVP. We find that if the initial population is equal to the two equilibrium points then the population remains at those values. Otherwise, below the smaller equilibrium point, the population decreases to zero and above the smaller equilibrium point, all solutions converge to the larger equilibrium point. Thus, we may conclude that if:

\(\displaystyle P(0)>\frac{kN-\sqrt{kN(kN-4H)}}{2k}\)

then:

\(\displaystyle \lim_{t\to\infty}P(t)=\frac{kN+\sqrt{kN(kN-4H)}}{2k}\)

Now, we may then write:

\(\displaystyle \lim_{H\to\frac{kN}{4}^{-}}\left(\frac{kN+\sqrt{kN(kN-4H)}}{2k} \right)=\frac{kN}{2k}=\frac{N}{2}\)

2.) If \(\displaystyle H>\frac{kN}{4}\) then there are no equilibrium points, and the parabolic frunction representing the slope of the solution will be negative for all values of $P$, which means that for any initial population value, the solution will decrease, hence:

\(\displaystyle \lim_{t\to\infty}P(t)=0\)

Recall we have:

\(\displaystyle \frac{dP}{dt}=kP\left(1-\frac{P}{N} \right)-H\)

Now, if we rewrite the right side in vertex form, we have:

\(\displaystyle \frac{dP}{dt}=-\frac{k}{N}\left(P-\frac{N}{2} \right)^2+\left(\frac{kN}{4}-H \right)\)

Thus, the largest values of \(\displaystyle \frac{dP}{dt}\) is:

\(\displaystyle \frac{kN}{4}-H\)

But, we have:

\(\displaystyle H>\frac{kN}{4}\implies \frac{kN}{4}-H<0\)

And so we have shown that the population will always decrease in this case, meaning that the population will always decline to zero.