Bobsled Impulse and Force -- ELASTIC collision problem

[sc8]

Homework Statement

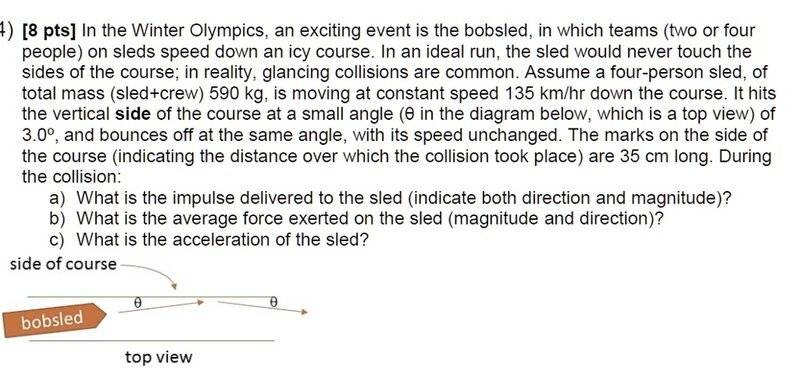
In the Winter Olympics, an exciting event is the bobsled, in which teams (two or four people) on sleds speed down an icy course In an ideal run; the sled would never touch the sides of the course; in reality, glancing collisions are common. Assume a four-person sled, of total mass (sled+crew) 590 kg; is moving at constant speed 135 km/hr down the course. It hits the vertical side of the course at a small angle (0 in the diagram below, which is a top view) of 3.00, and bounces off at the same angle, with its speed unchanged The marks on the side of the course (indicating the distance over which the collision took place) are 35 cm long: During the collision: a) What is the impulse delivered to the sled (indicate both direction and magnitude)? b) What is the average force exerted on the sled (magnitude and direction)? c) What is the acceleration of the sled? side of course top view bobsled

Relevant Equations

delta p=F*t

I attempted to do mvf-mvi to find the impulse, but had trouble figuring out what to use as v (where does the angle of 3degrees come in?), and thought that there had to be more to the problem considering the other details I was given. I then attempted to maybe calculate the kinetic energy lost, but on the exam it said that the collision was ELASTIC so I knew momentum AND kinetic energy had to be conserved.
Because this is elastic, would the momentum in the y direction just be reversed as the sled is moving slightly upwards at one point and down the other?
As for force, I was knew that distance had to come into play to find it, but I did not have time to get to that part before the exam finished. Would greatly appreciate if someone could help me understand how to approach this, thank you!

 

[hutchphd]

Yes the velocity is a vector as is the force.
How will you find t ?

 

[sc8]

Yes the velocity is a vector as is the force.
How will you find t ?

Well the force is delta p/ delta t... so I need the time and the change in momentum. I can find the time (I believe) from the distance of the collision, but the part I'm having trouble with is how to even calculate the impulse of the collision.

 

[hutchphd]

The equation you wrote down is correct and is true for any particular direction (i.e. it is a vector equation). So how do you calculate $\Delta {\vec p}$

 

[sc8]

Again, I already stated earlier in my attempted solution that I was unsure about what value I should use for v in the mf and mi calculations. I could not tell by the diagram which ratio of the angle to use for velocity.

 

[hutchphd]

The sled doesn't lose speed (by definition of problem) and it bounces off the wall. What must be the direction of the force?

 

[sc8]

Not following? If a force exerted by the sled onto the side of the course is Fab, then the force back from the side of the course would be -Fba.
I was asking for help how to approach this problem, so if you or someone else would actually care to explain how to solve it that would be helpful.

 

[hutchphd]

What is the direction of -Fba? That will cause the change in v. Do you understand vectors? What is your text for this course?

 

[sc8]

Can someone else please provide some help on this problem? I really want to understand how to solve!

 

[hmmm27]

The sled hits the side of the wall at a known speed* and a known angle. It bounces off the wall at a known (identical) speed and a known (mirror) angle. The contact distance is known, and the collision is completely elastic.

You're supposed to find a) impulse b) force(average) and c) acceleration. Why don't you work out what kind of calcs you need to do, and show some work towards solving them ; maybe some intermediate results.

* sortof : the wording says "down the course", without bothering to say if the ref frame is the centerline of the course, or the path of the bobsled (3deg off at time of collision).

 

[haruspex]

Can someone else please provide some help on this problem? I really want to understand how to solve!

The sled hits the side with a certain velocity. Velocity is a vector; it has magnitude (speed) and direction.
It bounces off with the same speed but a different direction. The impulse it got from the collision has caused a change in velocity. You need to calculate that change.
Do you understand how to add and subtract vectors?