Bose-Einstein Condensate Properties

[FallenApple]

So a Bose Einstein condensate is another state of matter at temperatures below those where a solid state exists.

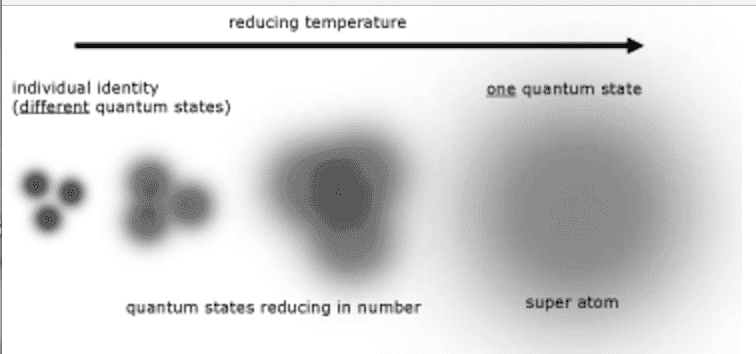
The temperature is reduced so much, that the quantum wave states overlap and become one single object.

So what are the properties of this object? Is it more rigid than solids?

Also, does it stay this way after the atoms have become one, even after the temperature goes up? If so, how? Conceptually, raising the temp should be different in solid to liquid to gas since those are simply aggregates of atoms, whereas a BEC is completely different. Are the identities of each component atom erased once it becomes part of the Bose Einstein condenstate?

What about the quarks and electrons, do they merge as well?

 

[DrClaude]

So what are the properties of this object? Is it more rigid than solids?

Definitely not. It is a superfluid.

Also, does it stay this way after the atoms have become one, even after the temperature goes up? If so, how?

In reality, you never get a pure condensate at T = 0K. There is a condensed fraction, with thermal atoms also present.

Conceptually, raising the temp should be different in solid to liquid to gas since those are simply aggregates of atoms, whereas a BEC is completely different.

You can't simply put a BEC in the s-l-g hierarchy, because it is not a stable state of matter. At the temperatures at which condensation occurs, the stable state would be solid. This is why dilute gases need to be used, to limit 3-body collisions that would lead to the formation of a solid.

Are the identities of each component atom erased once it becomes part of the Bose Einstein condenstate?

What about the quarks and electrons, do they merge as well?

You still have individual atoms, but they are fundamentally indistinguishable, and they occupy the same state and behave coherently. There is no "merging" taking place.

 

[FallenApple]

Definitely not. It is a superfluid.

Interesting. So I'm guessing that for a solid to exists, more pressure needs to be applied, and more pressure means more temperature.

You can't simply put a BEC in the s-l-g hierarchy, because it is not a stable state of matter. At the temperatures at which condensation occurs, the stable state would be solid. This is why dilute gases need to be used, to limit 3-body collisions that would lead to the formation of a solid.

Makes sense. Why would it be classified as another state of matter if it's just a special case of a liquid?

You still have individual atoms, but they are fundamentally indistinguishable, and they occupy the same state and behave coherently. There is no "merging" taking place.

So they are indistinguishable from each other? So they are all behaving in the same exact way, basically in unison?

 

[DrClaude]

Interesting. So I'm guessing that for a solid to exists, more pressure needs to be applied, and more pressure means more temperature.

It has nothing to do with pressure. When two identical atoms collide, because of conservation of energy, the only possible outcome is that the two atoms fly away from each other. For them to stick together, energy needs to be removed, which usually happens through a three-body collision, with the third atom carrying the extra energy.

In a sense, this is similar to the process of regular condensation, such as in the atmosphere, where a nucleation seed is needed for a rain drop or a snow flake to form.

Makes sense. Why would it be classified as another state of matter if it's just a special case of a liquid?

Because it is a special case? A superfluid state is so different from a normal liquid state that we consider it as separate. It also comes about through a phase transition, and thus the passage from normal liquid to superfluid is not fundamentally different from the passage from liquid to gas. If you look at the phase diagram for helium, you will clearly see also the distinction between the normal liquid and superfluid phases.

(Note that you could have said the same thing about liquids as compared to gases: liquids are just a special case of gases, since both are fluids. Above the critical point, you even lose the distinction between liquid and gas.)

So they are indistinguishable from each other? So they are all behaving in the same exact way, basically in unison?

That's a simplified picture, but correct.

 

[FallenApple]

It has nothing to do with pressure. When two identical atoms collide, because of conservation of energy, the only possible outcome is that the two atoms fly away from each other. For them to stick together, energy needs to be removed, which usually happens through a three-body collision, with the third atom carrying the extra energy.

In a sense, this is similar to the process of regular condensation, such as in the atmosphere, where a nucleation seed is needed for a rain drop or a snow flake to form.

Interesting. Does pressure help facilitate the process of this? I can imagine higher pressures help increase the likelihood of 3 body collisions.

Also, are BECs more dense than solids?

 

[Xu Shuang]

Still can't wrap my head around this. How exactly do we characterize entities made of fermions as bosons? If we have a bunch of cooper pairs, and they become a condensate, what exactly is the state of system look like if we return to the characterization of a bunch of electrons?

 

[DrClaude]

Interesting. Does pressure help facilitate the process of this? I can imagine higher pressures help increase the likelihood of 3 body collisions.

Anything that increases 3-body collisions will make it more difficult to form a BEC. We usually don't think of it in terms of pressure, as all this is done with trapped atoms in vacuum chamber, as collisions with background gases need to be avoided (it heats up the atoms).

Also, are BECs more dense than solids?

No, they are very dilute. If you tried to make it dense, it would again form a solid.

 

[DrClaude]

Still can't wrap my head around this. How exactly do we characterize entities made of fermions as bosons?

What is important is the property of a system as a whole. Take a hydrogen atom, made up of a proton and an electron, two fermions. The Pauli principle tells you that the wave function must change sign under exchange of two identical fermions, and when you are exchanging two hydrogen atoms, you are actually exchanging two electrons and two protons. So one exchange (the atoms) corresponds to two minus sings that cancel each other out, so the total wave function doesn't change sign under the exchange, hence the composite particle is a boson.

If we have a bunch of cooper pairs, and they become a condensate, what exactly is the state of system look like if we return to the characterization of a bunch of electrons?

I am not familiar enough with superconductivity and the BCS model to answer that.

 

[radium]

In mean field BCS theory you have Hamiltonian which has pairing terms that do not conserve particle number. These tell you that there is some attraction causing electrons to pair. Most of the time you consider a bound state of two electrons with zero net momentum, but if the pair has a finite momentum, you get what is called a pair density wave (PDW). If you diagonalize the Hamiltonian by introducing new fermionic creation and annihilation operators, you will get a spectrum of noninteracting Bogoliubov quasiparticles (superpositions of electrons and holes) which will be gapped. Excitations in this spectrum correspond to breaking apart Cooper pairs. So in this system below the critical temperature you will have a fraction of the electrons bound in Cooper pairs reflected in the trial BCS wavefunction by the Bogoliubov coefficients. The gap is related to these coefficients and is determined self consistently from mean field equations. When the gap is zero you've reached the critical temperature and the fraction of Cooper pairs goes to zero.

 

[bob012345]

In mean field BCS theory you have Hamiltonian which has pairing terms that do not conserve particle number. These tell you that there is some attraction causing electrons to pair. Most of the time you consider a bound state of two electrons with zero net momentum, but if the pair has a finite momentum, you get what is called a pair density wave (PDW). If you diagonalize the Hamiltonian by introducing new fermionic creation and annihilation operators, you will get a spectrum of noninteracting Bogoliubov quasiparticles (superpositions of electrons and holes) which will be gapped. Excitations in this spectrum correspond to breaking apart Cooper pairs. So in this system below the critical temperature you will have a fraction of the electrons bound in Cooper pairs reflected in the trial BCS wavefunction by the Bogoliubov coefficients. The gap is related to these coefficients and is determined self consistently from mean field equations. When the gap is zero you've reached the critical temperature and the fraction of Cooper pairs goes to zero.

What does it mean to diagonalize the Hamiltonian in physical terms if you are doing an experiment? Thanks.

 

[DrClaude]

What does it mean to diagonalize the Hamiltonian in physical terms if you are doing an experiment? Thanks.

How do you observe Cooper pairs experimentally?

 

[bob012345]

How do you observe Cooper pairs experimentally?

Please briefly tell me how that measurement equates to "diagonalizing the Hamiltonian" which is a mathematical operation. I'm interested in how mathematical terms equate to experimental reality. Are you just saying that any measurement effectively represents a diagonalized Hamiltonian? Thanks.

BTW, I've always wondered. When a BEC is used to slow light, what happens to the momentum of the light? Thanks.

 

[radium]

You can measure the energy gap and also the critical temperature (which of course is related to the energy gap). You can also measure things like the London penetration depth and the critical magnetic field strength at which superconductivity vanishes.

Diagonalizing a Hamiltonian tells you the spectrum of low energy excitations.

Right now I am talking about BCS type I superconductors which are much simpler than high Tc superconductors. In BCS theory, superconductivity is mediated by an electron phonon interaction causing a distortion in the lattice and a net attraction between electrons. This happens because of something called Cooper instability at low temperatures (of order the Debye frequency) which causes the electron phonon interaction (and hence the ability to form bound states) to win over electron repulsion. Another important thing to note is that the pairing here is a bound state of electrons (of zero momentum for BCS) and that electrons aren't actually right next to each other (like a ballroom dancer analogy). They could be quite far away.

 

[bob012345]

You can measure the energy gap and also the critical temperature (which of course is related to the energy gap). You can also measure things like the London penetration depth and the critical magnetic field strength at which superconductivity vanishes.

Diagonalizing a Hamiltonian tells you the spectrum of low energy excitations.

Right now I am talking about BCS type I superconductors which are much simpler than high Tc superconductors. In BCS theory, superconductivity is mediated by an electron phonon interaction causing a distortion in the lattice and a net attraction between electrons. This happens because of something called Cooper instability at low temperatures (of order the Debye frequency) which causes the electron phonon interaction (and hence the ability to form bound states) to win over electron repulsion. Another important thing to note is that the pairing here is a bound state of electrons (of zero momentum for BCS) and that electrons aren't actually right next to each other (like a ballroom dancer analogy). They could be quite far away.

Thanks, Radium!

Any thoughts anyone about my photon momentum question in a BEC?

 

[DrClaude]

BTW, I've always wondered. When a BEC is used to slow light, what happens to the momentum of the light? Thanks.

It gets transferred to the atoms.

 

[bob012345]

It gets transferred to the atoms.

The real question is if the momentum of photons is enhanced or not as the speed of light slows to a crawl.
Since in a vacuum the momentum is E/c, if c is 1m/s in a BEC is the momentum enhanced or not? The reason I'm asking is this, if one could exchange the power of a beam of photons to momentum of the BEC at a near 1/1 ratio, one has an incredible rocket engine that uses very low amounts of fuel. Thanks.

 

[DrDu]

The real question is if the momentum of photons is enhanced or not as the speed of light slows to a crawl.
Since in a vacuum the momentum is E/c, if c is 1m/s in a BEC is the momentum enhanced or not? The reason I'm asking is this, if one could exchange the power of a beam of photons to momentum of the BEC at a near 1/1 ratio, one has an incredible rocket engine that uses very low amounts of fuel. Thanks.

Why should a BEC be special as far as momentum is concerned? In every solid or liquid, momentum of light is transferred ot the medium and, if you are interested in acceleration by a laser, the maximum transfer of momentum is obtained in simple reflection from a mirror, as the momentum of the photons is reversed, so that 2 times the momentum of the photon is transferred to the mirror.

 

[bob012345]

Why should a BEC be special as far as momentum is concerned? In every solid or liquid, momentum of light is transferred ot the medium and, if you are interested in acceleration by a laser, the maximum transfer of momentum is obtained in simple reflection from a mirror, as the momentum of the photons is reversed, so that 2 times the momentum of the photon is transferred to the mirror.

It's not so simple as the nature of momentum in a solid has been debated for a century. The main issue is that in the BEC, c can be slowed to virtually nothing. If one takes the energy/momentum relationship literally it implies as c goes to say 1 m/s, the momentum transferred equates to the energy, not E/(3x10^8). If the BEC could be engineered to carry away much larger momentums than typical photon momentums, a small amount of fuel, the BEC atoms, might provide a reasonable thrust in space, comparable to at least an ion engine and perhaps better. For example, if one could get Newtons of thrust per KW of power for micrograms of BEC atoms, one has a great engine.

 

[DrDu]

From what I remember, the low velocity of light is due to extreme deviations of dispersion from linearity, so your argument is moot.

 

[Dale]

the nature of momentum in a solid has been debated for a century.

The debate had been resolved https://www.researchgate.net/profile/Brandon_Kemp/publication/234850066_Resolution_of_the_Abraham-Minkowski_debate_Implications_for_the_electromagnetic_wave_theory_of_light_in_matter/links/0a85e536a487b8673b000000.pdf

For some reason, the controversy seems more appealing than the resolution, leaving the false impression that it is unresolved.

If the BEC could be engineered to carry away much larger momentums than typical photon momentums

You don't need BEC for that. Ordinary chemical rockets carry away larger momentum than typical photon momentum. Energy and momentum conservation still apply, but in principle you can analyze the trade off flexibly.

 

[bob012345]

The debate had been resolved https://www.researchgate.net/profile/Brandon_Kemp/publication/234850066_Resolution_of_the_Abraham-Minkowski_debate_Implications_for_the_electromagnetic_wave_theory_of_light_in_matter/links/0a85e536a487b8673b000000.pdf

For some reason, the controversy seems more appealing than the resolution, leaving the false impression that it is unresolved.

You don't need BEC for that. Ordinary chemical rockets carry away larger momentum than typical photon momentum. Energy and momentum conservation still apply, but in principle you can analyze the trade off flexibly.

Thanks for the answer. If I understand it correctly, the Minkowski momentum would be true if the entire volume of space were a BEC medium but if it's a local medium around the ship, the center of mass of medium and ship goes as the Abraham momentum. I'm still processing the concept.

So, whether it's a BEC or not, we need something else since ordinary rockets are so very limited by large fuel requirements. I'm thinking of alternatives to ion thrusters and such devices. Were not going to get to the stars with rockets as we know them. There are beam energy schemes such as Robert Forward's ideas with extremely big lasers and other ideas including photon recycling schemes which gets more momentum out of each photon by recycling it many times but at the expense if having mirrors along the way in space. An ion drive has small thrust but can outperform a normal rocket by orders of magnitude in the long run. Even something like the VASIMIR engine which is far more capable than ordinary rockets is only a small step forward. Other exotic physics concepts may be much better.

http://www.nextbigfuture.com/2017/02/vasimr-plasma-rocket-targeting-100.htmlAn alternative is to use lasers to accelerate ions. There is a lot of research into advanced compact accelerator technology and some of it may be applicable to super advanced, compact and efficient space drives.

 

[Dale]

This seems way off topic from the OP's question.

 

[bob012345]

This seems way off topic from the OP's question.

Ok, back to the original question then...

Definitely not. It is a superfluid.In reality, you never get a pure condensate at T = 0K. There is a condensed fraction, with thermal atoms also present.You can't simply put a BEC in the s-l-g hierarchy, because it is not a stable state of matter. At the temperatures at which condensation occurs, the stable state would be solid. This is why dilute gases need to be used, to limit 3-body collisions that would lead to the formation of a solid.You still have individual atoms, but they are fundamentally indistinguishable, and they occupy the same state and behave coherently. There is no "merging" taking place.

What does it mean to say the BEC atoms all occupy the same state when there is definite size to the BEC, not like when Bosons occupy the same state they literally can occupy the same physical space, like N photons. At least that's what I was taught. If the atoms retain individuality it can be said that they have the same quantum numbers but they are not in the same state. Being physically separate defines a different state does it not? Especially if one could in principle follow one atom. I'm taking being in the same state as literally being in the same state. Also, consider band structure in semiconductors, there is a merging of the individual states into a communal band. Why should we not say the same of a BEC? Thanks.

 

[DrClaude]

What does it mean to say the BEC atoms all occupy the same state when there is definite size to the BEC, not like when Bosons occupy the same state they literally can occupy the same physical space, like N photons. At least that's what I was taught. If the atoms retain individuality it can be said that they have the same quantum numbers but they are not in the same state.

But they do occupy the same state. The fact that this state has a large physical extension has nothing to do with the fact that it is made up of many atoms, but is just the spatial extent of the ground state of the trapping potential.

Also, consider band structure in semiconductors, there is a merging of the individual states into a communal band. Why should we not say the same of a BEC?

Because we are not talking about states, but about population of states. Before and after BEC, the states are the same. The phase transition corresponds to a sudden macroscopic occupation of the ground state.

 

[DrDu]

If the atoms retain individuality [...] Especially if one could in principle follow one atom.

This is the main differerence between QM and classical mechanics. Atoms are no longer distinguishable, as, due to the Heisenberg principle, we can't follow their paths.

 

[bob012345]

This is the main differerence between QM and classical mechanics. Atoms are no longer distinguishable, as, due to the Heisenberg principle, we can't follow their paths.

Thanks. Based on a 20nK temperature of Rubidium atoms in a BEC, the HUP give an uncertainty in a nuclear position of about 0.15 microns. Given the extent of the BEC can be on the order thousands to millions of cubic microns it suggests they could be tracked in principle.

 

[bob012345]

But they do occupy the same state. The fact that this state has a large physical extension has nothing to do with the fact that it is made up of many atoms, but is just the spatial extent of the ground state of the trapping potential.

Thanks for the answer. I would say the electrons do occupy the same state but not the nuclei of the atoms and thus not the atoms as a whole. At least that's my interpretation.

 

[DrClaude]

Thanks. Based on a 20nK temperature of Rubidium atoms in a BEC, the HUP give an uncertainty in a nuclear position of about 0.15 microns. Given the extent of the BEC can be on the order thousands to millions of cubic microns it suggests they could be tracked in principle.

I don't know what you calculated there, but this has nothing to do with the HUP. The extent of the spatial wave function of each atom depends on the size of the trap (think "particle in a box," with all atoms in the ground state).

Thanks for the answer. I would say the electrons do occupy the same state but not the nuclei of the atoms and thus not the atoms as a whole. At least that's my interpretation.

Electrons are fermions, so I don't see how they could all occupy the same state by themselves. It is the atom as a whole that is a boson and the atoms in the BE-condensed phase are all in the same state. Your interpretation is incorrect.

 

[DrDu]

Thanks. Based on a 20nK temperature of Rubidium atoms in a BEC, the HUP give an uncertainty in a nuclear position of about 0.15 microns. Given the extent of the BEC can be on the order thousands to millions of cubic microns it suggests they could be tracked in principle.

But a volume of 0.15 10^3 cubic microns will still contain millions of atoms!

 

[bob012345]

But a volume of 0.15 10^3 cubic microns will still contain millions of atoms!

A volume of 0.15E3 cubic microns at typical BEC density of 1E13 atoms/cubic cm is 1500 contains atoms. I'm assuming a volume of say 30 cubic microns on a side or 2.7E4 um^3 which contains 2.7E5 atoms. That's a lot but the space contains ( 30/.15)^3 grid points which is 8E6
points. The 0.15um was the HUP uncertainty in position for that temperature and Rubidium atoms.