chwala
Gold Member
- 2,827
- 415
- Homework Statement
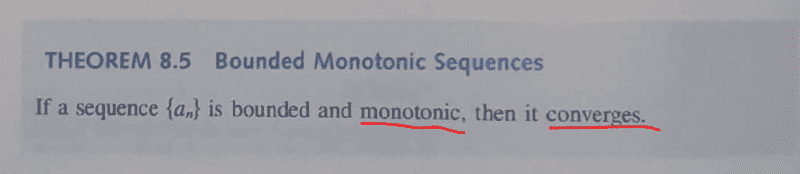
- see attached
- Relevant Equations
- Bounded and monotonic sequences - Convergence
I would like some clarity on the highlighted part. My question is, consider the the attached example ##(c)##, This sequence converges ( by using L'Hopital's rule)...now my question is, the sequence is indicated on text as not being monotonic...very clear. Does it imply that if a sequence is not monotonic, then it would not converge? In any case, what is the importance or rather the relevance of determining whether a sequence is monotonic (finding upper bounds and lower bounds) or not? Why not just take limit for the sequence and have the job done?