Bragg angle with an inclined plane through a square lattice

[LCSphysicist]

Homework Statement

.

Relevant Equations

.

Suppose a square lattice. The planes are such as the image below:

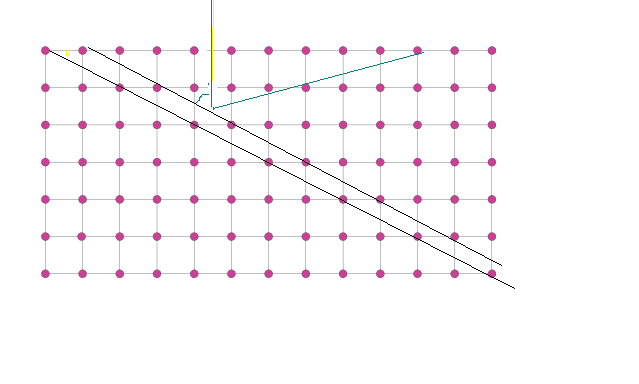
I light wave incides perpendicular to the square lattice.
The first maximum occurs for bragg angle (angle with the plane (griding angle) as $\theta_B = 30°$ (blue/green), green/blue in the figure).
The angle that the interplanes of the crystal make with the horizontal is $\alpha$ (yellow).
What is the de Broglie wave?

To be honest, i am so confused with this question that i have no idea what to say.

The first immediate confusion is how an ray coming perpendicularly to the crystal makes an angle $30°$, so that the complementar of it be $60°$, if obviously the angle (yellow) makes is $atan(d/2d) \neq 60°$

 

[Steve4Physics]

There appears to be a problem with the question/diagrams. Can you post the *exact* original question – word-for-word, with any supplied digrams? We might be able to help then.

By the way, the Bragg angle, $\theta_B$, is the glancing (not ‘griding’!) angle, i.e. the angle between a ray and the ‘reflecting’ plane.

 

[LCSphysicist]

There appears to be a problem with the question/diagrams. Can you post the *exact* original question – word-for-word, with any supplied digrams? We might be able to help then.

By the way, the Bragg angle, $\theta_B$, is the glancing (not ‘griding’!) angle, i.e. the angle between a ray and the ‘reflecting’ plane.

Yes, there indeed seems to appears a problem with the question.

Unfortunatelly, i can't post the image of the question for two reasons:
Another language
It was from a test* (so tecnically it is an official document? have no idea about laws)

But i will translate it for you:

"Consider, as the figure below, electrons hitting the cristal surface at right angles, and suffering diffraction of first order in an Bragg Angle $\theta_B = 30°$. Given that the atomic separation is $D =2.15 A°$, and that the lattice is a square lattice, calculate..."

What botters me is that we need the figure to know the plane he is talking about, but the figure is wrong.

Since it was from a test, i will try to contact the professor. But i am afraid i am the only one that notice that (I mean, not because i am special, but because if you just look at the figure to know which plane you should consider (diagonal? horizontal? etc), and ignore the angles at the figure, you can solve it) (obviously i assumed that i was wrong at the moment and was afraid to ask to professor)

The figure is equal the one i posted here.

 

[Steve4Physics]

I can't see how the question makes sense. If you find out, I (and probably others) would be interested if you could post the explanation.

 

[LCSphysicist]

I can't see how the question makes sense. If you find out, I (and probably others) would be interested if you could post the explanation.

The professor answered me. Indeed it is wrong/ambiguous.
Unfortunatelly, he will not cancel it, but will consider two answers possible (one in which $\alpha = 60°$ (and so the angle bragg is $30°$) and the other with $\alpha = \arctan(1/2)$, which by itself changes the whole question, so that the electrons incides not perpendicular anymore). Since i was not able to concluded which of the two reasoning were right at the moment of the test, my answer is wrong anyway.

So, let's reformulate the question so that it be more general? Even so i am extremelly sad, i am also curious what is the answer for more general cases.

Let the planar angle with the horizontal be $\alpha$, and the glancing angle corresponding to the maximum be $\theta$. What is the corresponding wavelength?
If you could give me a hit i would appreciate, i am not being able to wonder too much at the moment due to situation, but i will try later.

 

[Steve4Physics]

The professor answered me. Indeed it is wrong/ambiguous.
Unfortunatelly, he will not cancel it, but will consider two answers possible (one in which $\alpha = 60°$ (and so the angle bragg is $30°$) and the other with $\alpha = \arctan(1/2)$, which by itself changes the whole question, so that the electrons incides not perpendicular anymore). Since i was not able to concluded which of the two reasoning were right at the moment of the test, my answer is wrong anyway.

Thanks for confirming the question is wrong.

I don’t agree with your professor’s approach to handling an incorrect and infeasible problem - but that’s life!

So, let's reformulate the question so that it be more general? Even so i am extremelly sad, i am also curious what is the answer for more general cases.

Let the planar angle with the horizontal be $\alpha$, and the glancing angle corresponding to the maximum be $\theta$. What is the corresponding wavelength?
If you could give me a hit i would appreciate, i am not being able to wonder too much at the moment due to situation, but i will try later.

Let’s use:
interatomic spacing = D
plane spacing (distance between adjacent planes) = d

Some questions:

Q1. Express d in terms of D and $\alpha$

Q2. Knowing the Bragg angle ($\theta$) what is the standard formula relating $\lambda, \theta$, n and d for the n-th order maximum?

Q3. Use your answers to Q1 and Q2 to give the formula for $\lambda$.

 

[LCSphysicist]

Thanks for confirming the question is wrong.

I don’t agree with your professor’s approach to handling an incorrect and infeasible problem - but that’s life!Let’s use:
interatomic spacing = D
plane spacing (distance between adjacent planes) = d

Some questions:

Q1. Express d in terms of D and $\alpha$

Q2. Knowing the Bragg angle ($\theta$) what is the standard formula relating $\lambda, \theta$, n and d for the n-th order maximum?

Q3. Use your answers to Q1 and Q2 to give the formula for $\lambda$.

Yes, indeed. That what exactly what i have written at the test, just before realized the inconsistence between the angles, erase it, and derive a nonsense formula (trying to considere both cases to be true (90³ and 60³), which is impossible and proves that i was not under my sanity anymore LMAO).
By the way, the question here was solved so. The topic can be closed. THank you :)