British physics olympiad problem: A ball bearing bouncing off a steel cylinder

[hdp]

Homework Statement

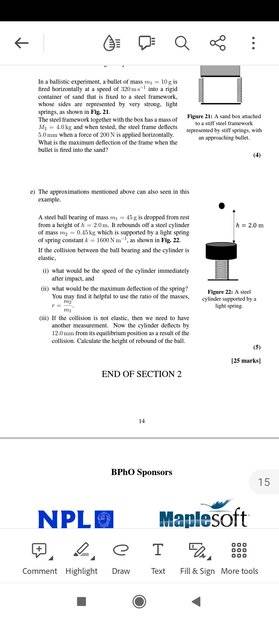
A steel ball bearing of mass m1 = 45 g is dropped from rest
from a height of h = 2.0 m. It rebounds off a steel cylinder
of mass m2 = 0.45 kg which is supported by a light spring
of spring constant k = 1600 N m−1
, as shown in Fig. 22.
If the collision between the ball bearing and the cylinder is
elastic,
(i) what would be the speed of the cylinder immediately
after impact, and
(ii) what would be the maximum deflection of the spring?
You may find it helpful to use the ratio of the masses,
r =m2/m1

Relevant Equations

Energy Conservation
Spring potential = 1/2 kx^2

I am struggling to find correct approximation for the problem. Is momentum conserved at the immediate impact of... (1)
Can I ignore gravitational force and potential for the spring which is connected to ground and vertically upholding a mass . ( using equilibrium)

 

[haruspex]

You will have to make an assumption about the spring constant for the collision between the ball and the cylinder. I would take it as arbitrarily large. That means you can consider that bounce as happening in an arbitrarily short time, before the spring gets further compressed at all.

 

[pbuk]

I am struggling to find correct approximation for the problem.

I think that may be because you are trying too hard. Read again the hints in the paper (unreadable in your image but quoted from the original at https://www.bpho.org.uk/Papers/R1/2021Nov_R1_S2.pdf):

Approximations and making a simple model are key to doing physics. Often we require linear behaviour in
our model, often associated with a small displacement from equilibrium, and also that the collision time is
short compared to any other motion of the system.

I like to think of this as (misquoting somebody) "everything should be made as simple as possible, but no simpler".

Is momentum conserved at the immediate impact of...

Why shouldn't it be? Would it be possible to answer part (i) if it wasn't?

Can I ignore gravitational force and potential for the spring which is connected to ground and vertically upholding a mass.

The question says the spring is light so what do you think? As you have no value for the mass of the spring would it be possible to answer the question if you couldn't ignore it?