Slimy0233
- 167
- 48
- Homework Statement
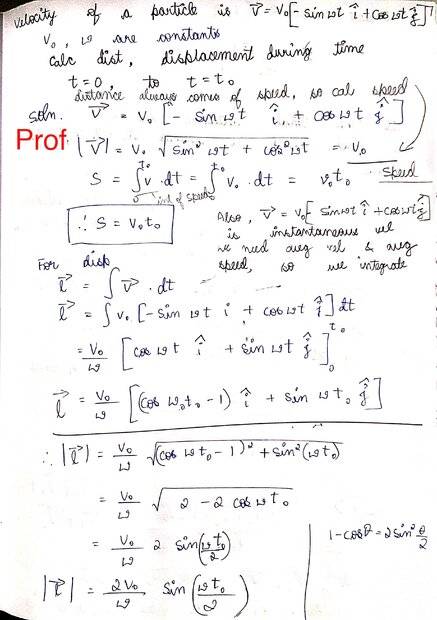
- Velocity of a particle is ##\vec{v}=V_0\left[-\sin \omega t \hat{i}+\cos \omega t \hat{j}\right]##
##V_0, \omega## are constants.
Calc dist, displacement during time ##t = 0## to ##t = t_{0}##
- Relevant Equations
- ##\vec{v}=V_0\left[-\sin \omega t \hat{i}+\cos \omega t \hat{j} \right]##
edit: I don't know why my latex isn't rendering, any help would be appreciated.
Edit 2: The question was due to a misunderstanding I had, I thought integrating instantaneous velocity would give me average velocity.
I have attached what I have tried so far. I had a doubt. Can you calculate the distance travelled by an object/particle using only the instantaneous velocity?
I mean, is the speed travelled by an object in time interval t = 0 to t = ##t_{0}##
S = ##V_{inst}*t_{0}##
My professor basically did this [Image with prof in it]
The other one is what I think the answer would be. I calculate the average velocity from instantaneous velocity by integrating ##V_{inst}## and then found out the average speed by finding the magnitude of the average velocity <vec{v}>
So, who is right? (I know I probably am wrong, but I want to know why)

Edit 2: The question was due to a misunderstanding I had, I thought integrating instantaneous velocity would give me average velocity.
I have attached what I have tried so far. I had a doubt. Can you calculate the distance travelled by an object/particle using only the instantaneous velocity?
I mean, is the speed travelled by an object in time interval t = 0 to t = ##t_{0}##
S = ##V_{inst}*t_{0}##
My professor basically did this [Image with prof in it]
The other one is what I think the answer would be. I calculate the average velocity from instantaneous velocity by integrating ##V_{inst}## and then found out the average speed by finding the magnitude of the average velocity <vec{v}>
So, who is right? (I know I probably am wrong, but I want to know why)
Last edited: