Calculate Max Resistance for 2 Parallel Wires

[Lambda96]

Homework Statement

How must the lengths $L_1$ and $L2$ be distributed so that the resistance between the points $X$ and $Y$ becomes maximum

Relevant Equations

none

Hi,

I am not sure if I have calculated the task here correctly:

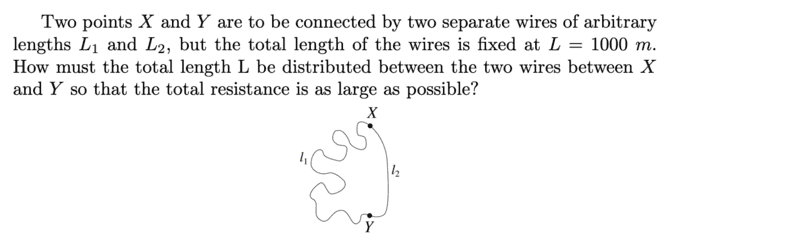
Based on the drawing, I now assumed that the two resistors are connected in parallel. The total resistance can then be calculated as follows $\frac{1}{R_T}=\frac{1}{R_1}+\frac{1}{R_2}$.

Since the two wires are made of the same material, I only considered their length in the calculation. Then I specified the following $L_1=x$ and $L_2=1000-x$ and substituted into the equation above $\frac{1}{x}+\frac{1}{1000-x}$

Since the resistance is supposed to be maximum, I calculated the derivative of the above expression and then set it to zero.

$$\frac{1}{(1000-x)^2}-\frac{1}{x^2}=0$$

After that, I simply solved the equation for x and got for $x=500m$ which gives me the following for the lengths $L_1=500m$ and $L_2=500m$.

 

[vela]

Looks fine, but you should verify you found a maximum rather than a minimum.

 

[Steve4Physics]

Then I specified the following $L_1=x$ and $L_2=1000-x$ and substituted into the equation above $\frac{1}{x}+\frac{1}{1000-x}$

I think you have treated the above expression as the total resistance. But it is not - it is the inverse of the total resistance.

Also, a good way of checking answers (when practical) is to compare the answer against extreme cases. E.g. what resistance would you expect if $L_1=L_2$? What resistance would you expect if $L_1= 1000m$ and $L_2=0$?

 

[jbriggs444]

I think you have treated the above expression as the total resistance. But it is not - it is the inverse of the total resistance.

Of course, since $f(x) = \frac{1}{x}$ is strictly monotone decreasing (barring the boundary where $x = 0$), searching for an extremum on $\frac{1}{x}$ is largely equivalent to searching for an extremum on $x$.

 

[Steve4Physics]

Of course, since $f(x) = \frac{1}{x}$ is strictly monotone decreasing (barring the boundary where $x = 0$), searching for an extremum on $\frac{1}{x}$ is largely equivalent to searching for an extremum on $x$.

Yes. Finding the extremum of $\frac 1{R_T}$ (which is a minmum here) is equivalent to finding the maximum of $R_T$.

But it's not clear (to me anyway) if that's what the OP has intentionally done!

 

[kuruman]

Isn't it easier to find an expression for the equivalent resistance and just look at it?
$$\frac{1}{R_{eq}}=\kappa\left(\frac{1}{L-x}+\frac{1}{x}\right)=\frac{\kappa~L}{x(L-x)}\implies R_{eq}=\frac{1}{\kappa}x(L-x).$$ This is equivalent to the problem of having a string of length $L$ and being asked to find the rectangle of perimeter $L$ that has maximum area. The answer is a rectangle of equal sides because for every rectangle of base $b$ and height $h$ there is another rectangle of base $h$ and height $b$ that has equal area. Thus, any $b\neq h$ is not unique and cannot be an extremum. Only $b=h$ gives a unique area and hence an extremum. It is a maximum because when either one of the sides goes to zero, the area goes to zero.

 

[kuruman]

Looks fine, but you should verify you found a maximum rather than a minimum.

If you are referring to the expression that OP is optimizing, it better have a minimum because it is the inverse of the equivalent resistance.

 

[Lambda96]

Thank you vela, Steve4Physics, jbriggs444 and kuruman, for your help and for checking my calculation