patric44
- 308
- 40
- Homework Statement
- calculate the average force on the face "y"
- Relevant Equations
- F = 1/2 rho*g*h*A
Hi All, in the following problem:

the book solution
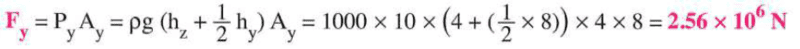
I don't understand why he added the term
$$
\rho g h_{z} A_{y}
$$
shouldn't it just be :
$$
F = 1/2 \rho g h_{y} A_{y}
$$
the book solution
I don't understand why he added the term
$$
\rho g h_{z} A_{y}
$$
shouldn't it just be :
$$
F = 1/2 \rho g h_{y} A_{y}
$$