Calculate the Magnetic Vector Potential of a circular loop carrying a current

[casparov]

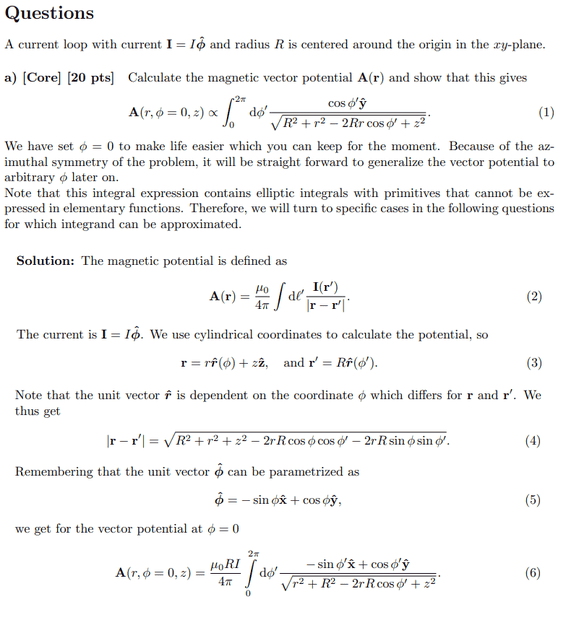
Homework Statement

Calculate the magnetic vector potential of a circular loop carrying a current

Relevant Equations

magnetic potential, cylindrical coordinates

Can someone explain what exactly happens at (4) ? I do not clearly follow, except that there is some cosine law going on?

I also do not really understand why at (3), r' doesnt have a z hat component, but I can live with that.

 

[hutchphd]

You need to realize that the current I(r') is nonzero on a loop in the xy plane at radius R. This limits the integration and provides symmetry.

 

[casparov]

You need to realize that the current I(r') is nonzero on a loop in the xy plane at radius R. This limits the integration and provides symmetry.

I thought it was due to symmetry, just a bit confused why we keep it in the unprimed, but I guess it is part of the definition of the vector in cylindrical system.

Can you please be able to explain how step 4 is achieved ?

 

[hutchphd]

You need to realize that the current I(r') is nonzero on a loop in the xy plane at radius R.

Then write out the denominator as a dot product.

 

[casparov]

Then write out the denominator as a dot product.

But it is not really a dot product is it ?

If I do that then, I get just the cosines right, and not the sines part also then ?

I guess my confusion lies at this position vector stuff, I really do not grasp it well.

 

[vela]

But it is not really a dot product is it ?

If I do that then, I get just the cosines right, and not the sines part also then ?

I guess my confusion lies at this position vector stuff, I really do not grasp it well.

The magnitude of a vector is the square root of the dot product of the vector with itself, so you have
$$\lvert \mathbf{r}-\mathbf{r'}| = \sqrt{(\mathbf{r}-\mathbf{r'})\cdot (\mathbf{r}-\mathbf{r'})}$$

 

[casparov]

The magnitude of a vector is the square root of the dot product of the vector with itself, so you have
$$\lvert \mathbf{r}-\mathbf{r'}| = \sqrt{(\mathbf{r}-\mathbf{r'})\cdot (\mathbf{r}-\mathbf{r'})}$$

Thank you very much for the reminder

 

[Steve4Physics]

Hi @casparov. It might be worth noting an alternative (but less elegant) approach - use Cartesian coordinates:

$\mathbf{r}= <r \cos \phi, r \sin \phi, z>$

$\mathbf{r’}= <R\cos \phi’, R \sin \phi’, 0>$

$| \mathbf{r}-\mathbf{r'}|^2 = (r \cos \phi - R\cos \phi’)^2 + (r \sin \phi - R\sin \phi’)^2 + (z - 0)^2$

which easily simplifies to equation (4).

In some situations, using Cartesian coordinates might be a convenient choice.

 

[casparov]

@Steve4Physics Thank you so much sir !! That's really helpful