scherz0
- 6
- 1
I grok, am NOT asking about, the answers below. Rather, how can I calculate the final answer DIRECTLY, without division? I don't know why my Latex isn't rendering here? Please see https://math.codidact.com/posts/285679.
Orange underline
1. Unquestionably, $\color{#FFA500}{4 \times 3/2} = 3!$ But how can I construe 3! DIRECTLY WITHOUT DIVISION? What does 3! mean?
Here's my surmisal. Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly. You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
Red underline
2. Unquestionably, ${\color{red}{\dfrac{8 \times 7 \times 6}{3!}}}$ = 8 × 7. But how can I construe 8 × 7 DIRECTLY WITHOUT DIVISION ? What does 8 × 7 mean?
Here's my surmisal. You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be 8 × 7 × 6? Why isn't there 6?
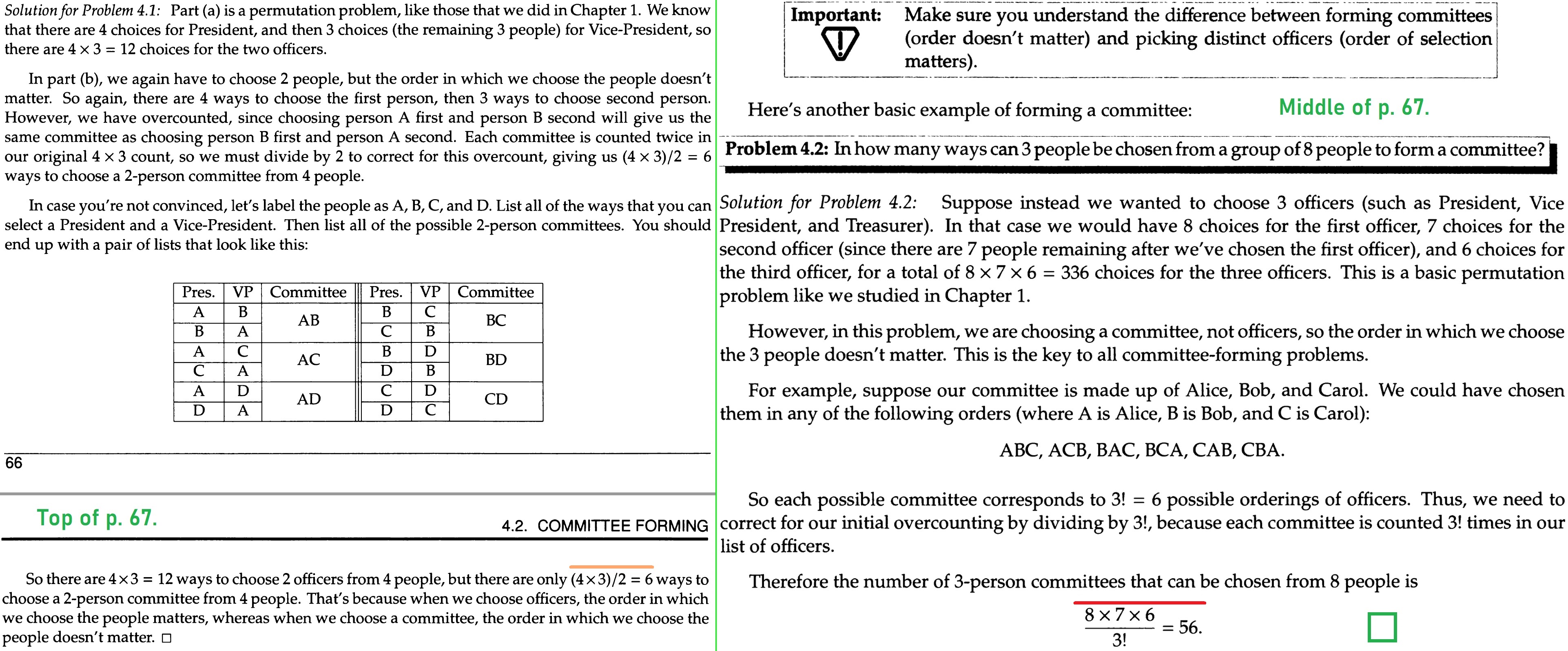
David Patrick, Introduction to Counting & Probability (2005), pp 66-7.
Orange underline
1. Unquestionably, $\color{#FFA500}{4 \times 3/2} = 3!$ But how can I construe 3! DIRECTLY WITHOUT DIVISION? What does 3! mean?
Here's my surmisal. Blitzstein's solution hints to this calculation, but he didn't write 3! explicitly. You fix the first person. Then of the 3 people left, you can pick the 2nd person in your 2-person committee in 3 ways. Is this correct?
Red underline
2. Unquestionably, ${\color{red}{\dfrac{8 \times 7 \times 6}{3!}}}$ = 8 × 7. But how can I construe 8 × 7 DIRECTLY WITHOUT DIVISION ? What does 8 × 7 mean?
Here's my surmisal. You can pick the 1st committee member in 8 ways, and the 2nd member in 7 ways. But then can't you pick the 3rd member in 6 ways? By this Constructive Counting (p 38 bottom), the answer ought be 8 × 7 × 6? Why isn't there 6?
Problem 4.1:
(b) In how many ways can a 2-person committee be chosen from a group of 4 people (where the order in which we choose the 2 people doesn't matter)?
David Patrick, Introduction to Counting & Probability (2005), pp 66-7.