Calculating Angular Acceleration for Link BD and ED: Verifying Solution

In summary, to find the angular acceleration of link BD and ED in a dynamics rigid body kinematics problem, the equations can be derived by assuming all links are of the same length (l). The angular velocity of link AB is given to be 45 rad/s CCW. The equations for the angular velocities of link BD and ED can be derived using the equations ωBD = ωAB - 2*π/n and ωED = ωBD + 2*π/n. Differentiating these equations with respect to time gives the equations for angular acceleration, which can be simplified to αED = αAB + 2*π/n. Since the angular velocity of link AB is constant, its angular acceleration is zero,
  • #1
grotiare
5
4
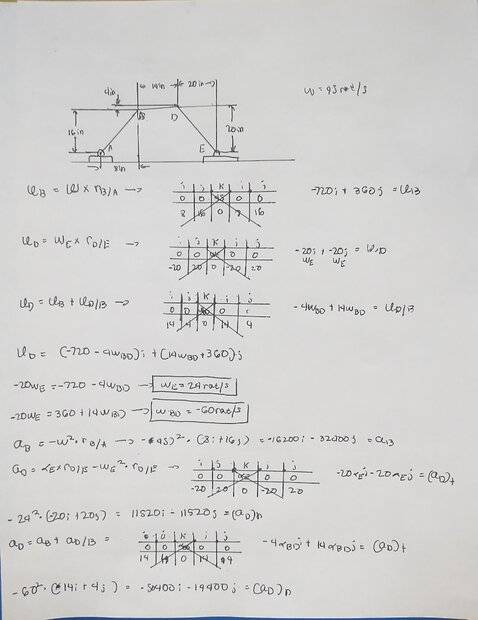
Homework Statement
angular acceleration of link BD and ED
Relevant Equations
Kinematics of rigid body formulas
Dynamics Rigid body Kinematics problem, looking for angular acceleration of link BD and ED. AB has constant angular velocity of 45 rad/s CCW. Could y'all verify any mistakes in my solution? Thanks!
IMG_20211211_095050302_HDR~3.jpg


IMG_20211211_095107988_HDR~2.jpg
 
Physics news on Phys.org
  • #2
Assuming that all links are of the same length (l), the equations for angular acceleration can be derived as follows:The angular velocity of link AB is given to be 45 rad/s CCW.The angular velocity of link BD is related to the angular velocity of link AB by the equation:ωBD = ωAB - 2*π/n (1)where n is the number of links in the chain. The angular velocity of link ED is related to the angular velocity of link BD by the equation:ωED = ωBD + 2*π/n (2)Differentiating Equation 1 with respect to time yields:αBD = αAB (3)Differentiating Equation 2 with respect to time yields:αED = αBD + 2*π/n (4)Substituting Equation 3 into Equation 4 yields:αED = αAB + 2*π/n (5)Since the angular velocity of link AB is constant, its angular acceleration is zero.Therefore, the angular acceleration of link BD is zero and the angular acceleration of link ED is 2*π/n.
 

FAQ: Calculating Angular Acceleration for Link BD and ED: Verifying Solution

How do you calculate angular acceleration for Link BD and ED?

To calculate angular acceleration for Link BD and ED, you need to use the formula: α = (ω2 - ω1) / (t2 - t1), where ω2 and ω1 are the final and initial angular velocities respectively, and t2 and t1 are the final and initial times respectively.

What is the unit of measurement for angular acceleration?

The unit of measurement for angular acceleration is radians per second squared (rad/s2).

How do you verify the solution for calculating angular acceleration?

To verify the solution for calculating angular acceleration, you can use the formula: α = Δω / Δt, where Δω is the change in angular velocity and Δt is the change in time. You can also check if the units of measurement are correct.

What factors can affect the accuracy of the calculated angular acceleration?

The accuracy of the calculated angular acceleration can be affected by factors such as measurement errors, friction, and external forces acting on the object.

Can angular acceleration be negative?

Yes, angular acceleration can be negative. A negative angular acceleration indicates that the object is slowing down or decelerating.

Similar threads

Angular Acceleration and relative velocity
Replies
5
Views
3K
Offset Piston - Calculating Max. Angular Velocity
Replies
5
Views
4K
What Are the Angular Velocity and Acceleration in This Mechanism?
Replies
7
Views
5K
Linking gravity, angular velocity and radius
Replies
5
Views
5K
Engineering Dynamics - Velocity and Acceleration Analysis
Replies
1
Views
3K
Linear acceleration, Angular acceleration, tension.
Replies
9
Views
5K
Maximizing Velocity and Acceleration in a Slider Crank Mechanism
Replies
2
Views
8K
Coriolis Acceleration of a Mechanism
Replies
4
Views
2K
Angular velocity and angular acceleration of a turbine
Replies
17
Views
2K
Planar Kinematics: Determine Angular Velocity of Plate BCD
Replies
2
Views
2K

Hot Threads

Recent Insights

Back
Top