hidemi
- 206
- 36
- Homework Statement
- A 0.20-kg particle moves along the x axis under the influence of a conservative force. The potential energy is given by
U(x) = (8.0 J/m^2)x^2 + (2.0 J/m^4)x^4,
where x is in coordinate of the particle. If the particle has a speed of 5.0 m/s when it is at x = 1.0 m, its speed when it is at the origin is:
a) 0 m/s
b) 2.5 m/s
c) 5.7 m/s
d) 7.9 m/s
e) 11 m/s
The answer is E.
- Relevant Equations
- K1 + U1 = K2 + U2
The correct answer can be obtained by the calculation as attached.
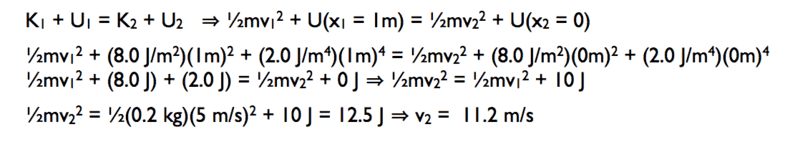
However it can not be gotten by the following way. Why?
F = -∇U = -[ 16x + 8x^3] = ma
Since m = 0.2, a = -80x - 40x^3
V = -40x^2 - 10x^4 +C =5
c= 50 + 5 =55
However it can not be gotten by the following way. Why?
F = -∇U = -[ 16x + 8x^3] = ma
Since m = 0.2, a = -80x - 40x^3
V = -40x^2 - 10x^4 +C =5
c= 50 + 5 =55