forces9912
- 6
- 3
- Homework Statement
- Problem in the images
- Relevant Equations
- mgh = 1/2 m v^2 + 1/2 I w^2 - Work
s = u t + 1/2 a t^2
a = v/t
I = 1/2 m R^2
I am stuck on what to do to calculate the inertia of a flywheel using the method described.
I am supposed to use conservation of energy equations to calculate the inertia.
I have a picture of the experiment and also the measurements I have taken.

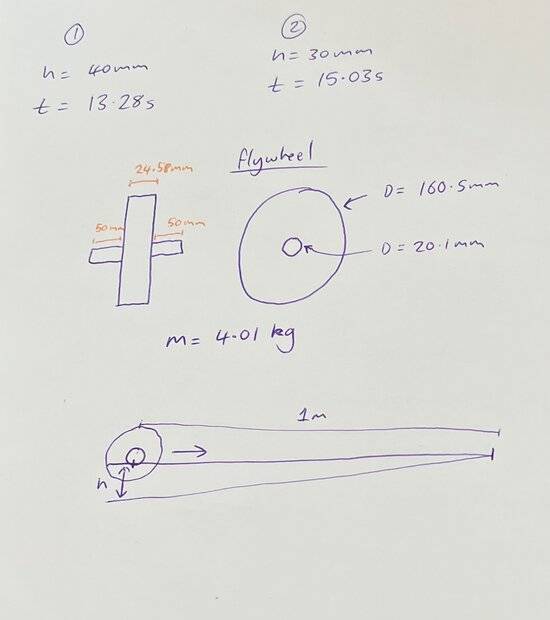 It seems each method I try I get a different number for Inertia.
It seems each method I try I get a different number for Inertia.
Any help on how to tackle this problem would be greatly appreciated.
I am supposed to use conservation of energy equations to calculate the inertia.
I have a picture of the experiment and also the measurements I have taken.
Any help on how to tackle this problem would be greatly appreciated.