Calculating Force Applied to Box with Friction Coefficient \mu

Click For Summary
SUMMARY

The discussion centers on calculating the force required to move a box with mass x on a surface with a friction coefficient μ. The participants clarify that the normal force (N) acting on the box is equal to the weight (W) of the box but acts in the opposite direction, ensuring no net vertical force. This understanding is crucial for accurately determining the applied force needed to overcome friction and achieve horizontal movement.

PREREQUISITES
  • Understanding of Newton's laws of motion
  • Familiarity with the concepts of friction and normal force
  • Basic knowledge of vector forces and their components
  • Ability to perform calculations involving mass, weight, and angles
NEXT STEPS
  • Study the relationship between frictional force and normal force in physics
  • Learn how to resolve forces into their components using trigonometry
  • Explore the implications of different friction coefficients on motion
  • Investigate applications of these principles in real-world scenarios, such as vehicle dynamics
USEFUL FOR

Physics students, engineers, and anyone interested in understanding the mechanics of motion and friction in practical applications.

Angello90
Messages
65
Reaction score
0
Hi guys,

I got this thought in my head.

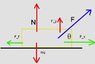
If I have a box of mass x, being pulled towards East and the angle [tex]\theta[/tex],
then force applied to the box in order to move it on the surface with friction coefficient [tex]\mu[/tex] has to be in such a way:

attachment.php?attachmentid=25494&stc=1&d=1272632393.jpg


My question is... wouldn't N be:

attachment.php?attachmentid=25496&stc=1&d=1272632659.jpg


Or, N would be as usual W but in opposite direction? The drawing shows all the forces on the box:

Cheers and thanks for the thought

attachment.php?attachmentid=25495&stc=1&d=1272632393.jpg
 

Attachments

  • 1.jpg
    1.jpg
    2.3 KB · Views: 509
  • 2.jpg
    2.jpg
    11.7 KB · Views: 514
  • 3.jpg
    3.jpg
    2.2 KB · Views: 657
Physics news on Phys.org
Angello90 said:
My question is... wouldn't N be:

attachment.php?attachmentid=25496&stc=1&d=1272632659.jpg
You got it.

Or, N would be as usual W but in opposite direction?
Nope. You were right the first time. The key is that there must be no net force on the box in the vertical direction.
 
Ok thanks a mil! I was bit confused
 

Similar threads

High School Are frictional forces action forces or reaction forces?
  • · Replies 14 ·
Replies
14
Views
2K
Undergrad If frictional force in system is <= us*N then there is no slipping
  • · Replies 7 ·
Replies
7
Views
2K
Undergrad Modeling Coulomb Friction for a Moving Point Mass in a Circular Bowl
  • · Replies 20 ·
Replies
20
Views
2K
High School Equivalence of Frictional and Applied Force
  • · Replies 2 ·
Replies
2
Views
2K
Undergrad Reaction force of and its relation to normal force and friction
  • · Replies 20 ·
Replies
20
Views
5K
Undergrad Determine if the block will slide or flip over after contact
  • · Replies 8 ·
Replies
8
Views
2K
Undergrad Is Mechanical Energy Conservation Free of Ambiguity - follow up
  • · Replies 77 ·
3
Replies
77
Views
6K
Undergrad What determines the direction of static friction forces?
  • · Replies 6 ·
Replies
6
Views
2K
Undergrad Calculate combined friction on multiple different surfaces?
  • · Replies 2 ·
Replies
2
Views
11K
Undergrad Will This Friction Clamp Hold the Weight?
  • · Replies 10 ·
Replies
10
Views
2K