Calculating 'g' Variation on Earth's Surface

[Sekonda]

Hey,

The question is displayed in the image below:

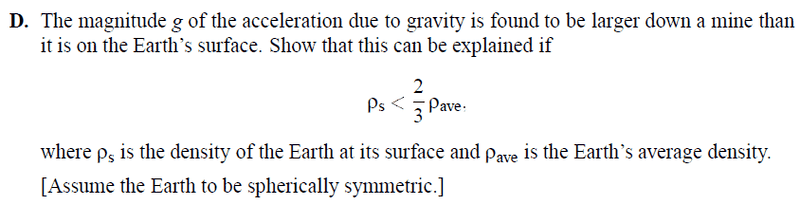
So I have approached this question using g=-GM/(r^2), for the surface and the Earth as a whole. Though when it talks about the average density I wasn't sure if it meant the whole Earth or a sphere at a smaller radius (that of the mine). Nonetheless I have tried it both ways using 'R-d' as the radius for the density of the average and just 'R' ('R' being the radius of the Earth).

I wasn't sure what I should use as the volume of the surface of the Earth and have tried using 4piR^2 dR and other values, I suppose that is my main question; what do I use as the volume of the Earth's surface?

Thanks!
Sorry if I'm not clear,
S

 

[D H]

This generalizes to $\rho(r) < \frac 2 3 \rho_{\text{ave}}(r)$, where $\rho(r)$ is the density of the rock at a distance r from the center of the Earth and $\rho_{\text{ave}}(r)$ is the average density of that part of the Earth that is inside a sphere with center at the center of the Earth and a radius of r. Gravitational acceleration inside the Earth increases with increasing depth if the local density is less than 2/3 the average density of all of the stuff inside that radius. (And decreases if the local density is at least 2/3 of that average density). What this means is that gravitational force increases with increasing depth in the Earth's crust because the average density of the Earth as a whole is 5.515 grams/cubic centimeter but rock in the Earth's crust has a density of about 2.7 grams/cubic centimeter, which is considerably less than 2/3 of the average density.


Your job is to show that this 2/3 ratio is the case.

Hint: Try to find $\partial g(r) / \partial r$.

 

[Sekonda]

I found dg/dr to be (-4pi/3)(rp'(r)-p(r))

where p'(r) is the derivative of p(r) with respects to r.

Is this correct,

Oh and thank you for your thorough explanation of the problem; I understand it more fluently now, just trying to describe it mathematically which seems to be the issue for me.

Thanks!

 

[tms]

I found dg/dr to be (-4pi/3)(rp'(r)-p(r))

where p'(r) is the derivative of p(r) with respects to r.

Is this correct,

I assume that by $p$ you mean $\rho$. What you need to do first is find the equation for $g$ as a function of $r$ and $\rho_{\text{ave}}$ (and whatever other variables are appropriate). You are not concerned with $\rho(r)$; you only need, and know, the average density and the surface density.

 

[Sekonda]

Right so I have the equation:

g(r,p(ave))=-4G*p(ave)*pi*r/3

is this correct and do I need to construct an equation for g(r,p(surface))?

 

[D H]

I found dg/dr to be (-4pi/3)(rp'(r)-p(r))

where p'(r) is the derivative of p(r) with respects to r.

Is this correct,

You have a sign error there that's going to trip you up.

This, from a later post is correct:

g(r,p(ave))=-4G*p(ave)*pi*r/3

Differentiate with respect to r and you'll get something similar to what you have above.

What you need to do to solve this problem is find dρ_ave/dr.

Hint: Express the M(r), the mass of that part of the Earth inside the radius r, as an integral that involves the instantaneous (not average) density ρ(R).

 

[tms]

What you need to do to solve this problem is find dρ_ave/dr.

My understanding is that $\rho_{\text{ave}}$ is constant. The change in average density after removing the shell above you should be insignificant if you don't go down too far.

 

[D H]

Right so I have the equation:

g(r,p(ave))=-4G*p(ave)*pi*r/3

Since you are worried about the magnitude of the gravitational force, there is no need for that minus sign on the front.

My understanding is that $\rho_{\text{ave}}$ is constant. The change in average density after removing the shell above you should be insignificant if you don't go down too far.

That's incorrect. It is the gradient in the average density that makes the magnitude of the gravitational force increase rather than decrease with increasing depth. Gravitational force would decrease with increasing depth if $\bar{\rho}$ was constant.

 

[tms]

That's incorrect. It is the gradient in the average density that makes the magnitude of the gravitational force increase rather than decrease with increasing depth. Gravitational force would decrease with increasing depth if $\bar{\rho}$ was constant.

I meant that in the context of this problem the average density should be considered constant. If not, how do you find ${\partial\rho(r)}/{\partial r}$ with the information given in the problem? Further, if you know $\rho(r)$, why do you need to worry about average density at all?

 

[D H]

I meant that in the context of this problem the average density should be considered constant.

It can't. The OP has correctly determined that
$$g(r) = \frac{GM(r)}{r^2} = \frac{G\frac 4 3 \pi \bar{\rho}(r) r^3}{r^2} = \frac 4 3 \pi G r \bar{\rho}(r)$$
If $\bar{\rho}(r)$, the average density of the mass within a sphere of radius r, is constant, then $dg(r)/dr = 4/3 \pi G \bar{\rho}$. In other words, with constant density, gravitational acceleration inside the Earth increases (at a constant rate) as radius increases, which of course means that gravitational acceleration decreases with increasing depth. The point of this problem is to show that gravitational acceleration increases with increasing depth, at least initially.

Aside: Going from the surface down toward the center of the Earth, gravitational acceleration increases initially, then starts decreasing at the transition zone between the upper and lower mantle, the starts increasing again inside the lower mantle to reach a maximum of 10.68 m/s² at the core/mantle boundary. Gravitational acceleration drops toward zero inside the core.

If not, how do you find ${\partial\rho(r)}/{\partial r}$ with the information given in the problem?

You use calculus.

Further, if you know $\rho(r)$, why do you need to worry about average density at all?

Because that is what the problem is asking for.

 

[Sekonda]

Ok so I need to determine M(r), would this be a correct integral:

∫4pi*(r^2)*p(r)dr

with limits of some point 'r' to '0'?

Thanks again guys

 

[D H]

Very good.

 

[D H]

Another hint:
$$\bar{\rho}(r) = \frac{M(r)}{4/3\pi r^3}$$
From this, what is $\frac{d\bar{\rho}(r)}{dr}$? You'll need to use your integral to compute $\frac{dM(r)}{dr}$.

 

[Sekonda]

Ok so I have :

$$\LARGE \frac{\partial \bar{\rho}(r)}{dr}=\frac{\frac{4\pi r^3 \bar{\rho}}{3}-M(r)}{\frac{4\pi^3 r^8}{9}}$$

Is this right? I presumed the derivative of M(r) was just equal to the term in the integral.

 

[D H]

Ok so I have :

$$\LARGE \frac{\partial \bar{\rho}(r)}{dr}=\frac{\frac{4\pi r^3 \bar{\rho}}{3}-M(r)}{\frac{4\pi^3 r^8}{9}}$$

Is this right? I presumed the derivative of M(r) was just equal to the term in the integral.

That's not right. Look at the units. The left hand side is density/length, or mass/length⁴. The right hand side has a numerator with units of mass (both terms) and a denominator with units of length⁸, so altogether, mass/length⁸. Your units don't match.

As far as the derivative of M(r), that $\rho(r)$ in the integral is not the average density $\bar{\rho}(r)$. It is the density of the rock at a distance r from the center of the Earth.

What does the fundamental theorem of calculus say about
$$\frac{d}{dr}\left(\int_0^r 4\pi\,\xi^2\rho(\xi)\,d\xi\right)$$

 

[Sekonda]

Ahh yes of course the density is not the average; and does the fundamental theorem of calculus imply that the derivative of that particular integral is:$$\LARGE 4\pi r^2 \rho(r)$$

I'm guessing we can choose r=Radius of Earth and the this gives us the density $$\LARGE \rho _{s}$$ ?

 

[D H]

Ahh yes of course the density is not the average; and does the fundamental theorem of calculus imply that the derivative of that particular integral is:


$$\LARGE 4\pi r^2 \rho(r)$$

Correct.

Now fix your derivative of $d\bar{\rho}(r)/dr$, and use this expression for $dM(r)/dr$ in the corrected derivative of $d\bar{\rho}(r)/dr$.

The ultimate goal is to come up with a simple expression (do it right and it will be very simple!) for $dg(r)/dr$. That of course is the derivative with respect to increasing radial distance. You want the derivative with respect to increasing depth. Getting this final derivative is a trivial transformation.

 

[Sekonda]

Right I've finally solved it, and the maths does look simpler now looking over it!

Thanks for the help, much appreciated!

S