Calculating non-dielectric reflectance without using complex numbers

[kaasisdebaas]

TL;DR

I need a to calculate the fresnel reflection ratio of a non dielectric material given the incident angle, the refractive indexes of the incident and interfacing materials and the extinction coefficient of the interfacing material. I need to to this without directly using complex numbers.

I need a to calculate the fresnel reflection ratio of a non dielectric material given the incident angle, the refractive indexes of the incident and interfacing materials and the extinction coefficient of the interfacing material. I need to to this without directly using complex numbers, due to limitations of the software i am using.

I do not have a physics background, but here is what i have been able to figure out so far.

given
incident angle θ_i in radians
incident IOR n₁
interfacing IOR n₂
and interfacing extinction coefficient k

I calculate the transmissive angle using snells law

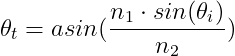
Then using the fresnel equations I calculate the effective reflectance

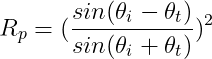
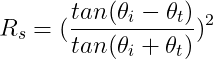
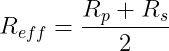
this gives me this graph for a air to glass interface which looks correct

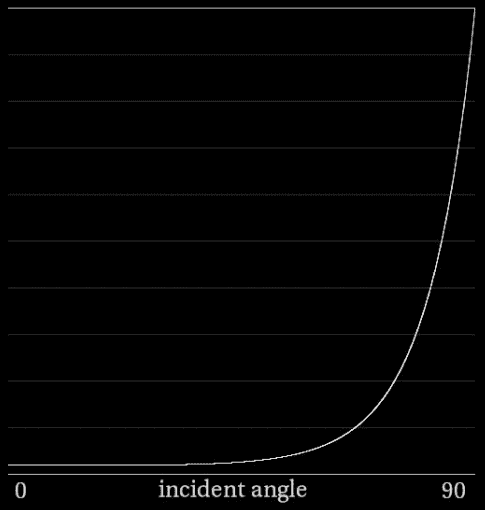
this works for dielectric materials that have a neglectable extinction coefficient, but for metals i need to extend the formulas i have. all the reference i can find extends the IOR with an imaginary component that handles the extinction coefficient, the problem is the graphic software im using does not allow complex numbers, so i need some way to extend the formulas i have using real numbers only.

the only reference i was able to find is this page https://seblagarde.wordpress.com/2013/04/29/memo-on-fresnel-equations/#more-1921
the code on that page is avoiding the use of complex numbers but i am unable to make heads or tails of what is actually happening here.
To be clear this is not the software im working in, this is just an example of an intergration that seems to not use complex numbers directly. I am just looking for the equations that describe it

any help intergrating the extinction coefficient into the existing formulas above is appreciated, thanks!

 

[anuttarasammyak]

Introducing imaginary number is helpful for calculation and we get real results we need at the end. I observe it in the page you refer.

 

[kaasisdebaas]

Introducing imaginary number is helpful for calculation and we get real results we need at the end. I observe it in the page you refer.

right, I understand that it is usefull, however the software i need this to work in does not allow for complex or imaginairy numbers, hence the question.

 

[Baluncore]

.... the only reference i was able to find is this page https://seblagarde.wordpress.com/2013/04/29/memo-on-fresnel-equations/#more-1921
the code on that page is avoiding the use of complex numbers but i am unable to make heads or tails of what is actually happening here.

I suspect that a "float3" structure is made from three floating-point real numbers. Those three values .x, .y, and .z, define a 3D point, or a vector in 3D space.

Before I could expand the float3 functions needed into 1D real arithmetic, I would need a reference to the float3 structure and library.
https://learn.microsoft.com/en-us/windows/win32/numerics_h/float3-structure

It may be easier to import the float3 library, then refer to the elements using the .x, .y or .z components, which are real numbers.

 

[kaasisdebaas]

I suspect that a "float3" structure is made from three floating-point real numbers. Those three values .x, .y, and .z, define a 3D point, or a vector in 3D space.

Before I could expand the float3 functions needed into 1D real arithmetic, I would need a reference to the float3 structure and library.
https://learn.microsoft.com/en-us/windows/win32/numerics_h/float3-structure

It may be easier to import the float3 library, then refer to the elements using the .x, .y or .z components, which are real numbers.

I edited the original post, cause there seems to be some confusion, this is not the software in using, its only an example i found that seems to not directly use complex numbers in its intergration.

 

[berkeman]

I edited the original post, cause there seems to be some confusion,

Just a point of discussion forum etiquette -- it's usally best to post a follow up reply with the corrections. Editing your OP after issues have been pointed out confuses all who read the thread afterward (since the issues are not there in your OP anymore).

 

[Baluncore]

... this works for dielectric materials that have a neglectable extinction coefficient, but for metals i need to extend the formulas i have. all the reference i can find extends the IOR with an imaginary component that handles the extinction coefficient, the problem is the graphic software im using does not allow complex numbers, so i need some way to extend the formulas i have using real numbers only.

Then you need to give us a copy of the code with the imaginary component using complex numbers. We can then expand that out into real arithmetic for you.