Calculating Pressure in a Flowing Liquid

[vcsharp2003]

Homework Statement

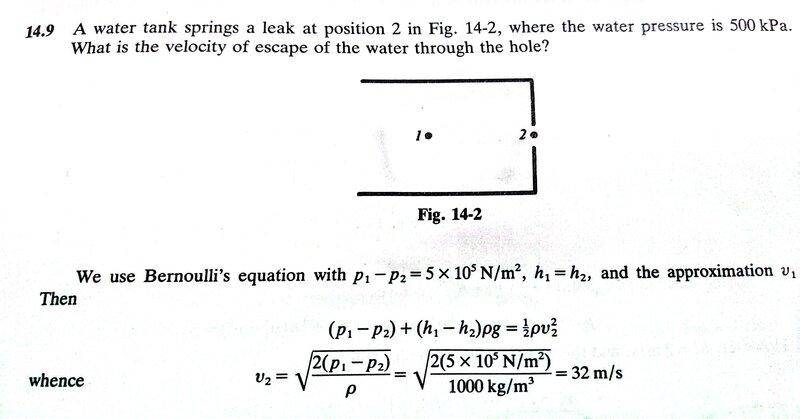
I was looking at a fluid mechanics problem from the Schaum's Outline Series book by the name of College Physics. The problem and its solution are as in the screenshot.
What I don't understand is why the water pressure given at 2 is actually $p_1-p_2$ and not $p_2$?

Relevant Equations

Bernoulli's equation
$p_1+\frac {1}{2} \rho {v_1}^2 +h_1 \rho g$
$=p_2+\frac {1}{2} \rho {v_2}^2 +h_2 \rho g$

According to what I get, the problem clearly says that $p_2= 500$ kPa. I can also see that $p_1 > p_2$ since higher velocity means lower pressure in a flowing liquid and we can say that $v_1<v_2$.

 

[kuruman]

The pressure at 2 is atmospheric. The pressure at 1 is presumably gauge pressure, not absolute. Therefore Δp = 500 kPa.

 

[vcsharp2003]

The pressure at 2 is atmospheric. The pressure at 1 is presumably gauge pressure, not absolute. Therefore Δp = 500 kPa.

But it says water pressure at 2 is 500 kPa and not at 2, unless I'm reading it wrong.

 

[kuruman]

The statment of the problem is badly phrased. The pressure inside the pipe, e.g. at point 1, the pressure is 500 kPa. At some point, the pressure drops to atmospheric when the fluid exits the pipe. That's point 2.

 

[Lnewqban]

On the left side of point 2, the static pressure must be the same as for point 1, as per Pascal’s principle: 500 kPa (not clear if gauge or absolute value).
As explained above, the static pressure on the right side of point 2 is 101.33 kPa (standard atmospheric pressure).

 

[vcsharp2003]

The statment of the problem is badly phrased. The pressure inside the pipe, e.g. at point 1, the pressure is 500 kPa. At some point, the pressure drops to atmospheric when the fluid exits the pipe. That's point 2.

That's what my initial expectation was. Anytime fluid is exposed to atmosphere, the fluid pressure at the exposed section must be $p_a$ i.e. atmospheric pressure. But then I thought that maybe point 2 is connected to a pipe and so pressure is 500 kPa in that small pipe at 2.

 

[vcsharp2003]

On the left side of point 2, the static pressure must be the same as for point 1, as per Pascal’s principle: 500 kPa (not clear if gauge or absolute value).
As explained above, the static pressure on the right side of point 2 is 101.33 kPa (standard atmospheric pressure).

The fluid is flowing and so we should not be using the static pressure formula $p = \rho h g$, but the pressure satisfying Bernoulli's equation. Right?

 

[Lnewqban]

It would not be like that for a real viscous fluid.
There would be contraction of flow vanes, turbulence, etc., inducing a pressure gradient around that sharp-edged-hole.

Leaving velocity would be less than calculated this way.
Those types of transitions are made intentionally smooth, with gradual reduction of the cross-section, and following a kind of conical shape.

 

[kuruman]

That's what my initial expectation was. Anytime fluid is exposed to atmosphere, the fluid pressure at the exposed section must be $p_a$ i.e. atmospheric pressure. But then I thought that maybe point 2 is connected to a pipe and so pressure is 500 kPa in that small pipe at 2.

Schaum's Outlines problems are not known for complexity. What you see, is what you get. Your initial expectation was on the mark.

 

[vcsharp2003]

Schaum's Outlines problems are not known for complexity. What you see, is what you get. Your initial expectation was on the mark.

I think this problem needs to be corrected in the book. It's a mistake in this popular book.

 

[haruspex]

I think this problem needs to be corrected in the book. It's a mistake in this popular book.

It's just sloppy wording. It means "where the pressure had been …", i.e. before the leak. It should also specify gauge pressure.

 

[vcsharp2003]

It's just sloppy wording. It means "where the pressure had been …", i.e. before the leak. It should also specify gauge pressure.

This is the first time I have come across an error in a Schaum's Series book. Schaum's Series books are impeccable and perfect in all subjects and so easy to understand and learn from, unlike the textbooks.

 

[vcsharp2003]

It's just sloppy wording. It means "where the pressure had been …", i.e. before the leak. It should also specify gauge pressure.

That might make sense. The problem statement might not have an error.

The term "water pressure" in the question could mean the pressure due to the water column above it i.e due to $\rho h g$. Using this fact, I have presented my take on this question as below.

The pressure at point 2 was (water pressure + atmospheric pressure) i.e. 500 kPa + $p_a$ before the sudden leak started. Now we know that in a static fluid the pressures at points having the same height of liquid above them are equipressure points; therefore the pressure at point 1 was also 500 kPa + $p_a$ just before the leak.

When the leak suddenly started we have no more a static fluid scenario but a flowing fluid scenario. The pressure at point 2 now changes to atmospheric pressure i.e $p_a$ since it's in contact with atmosphere. Now, at point 1 we are assuming $v_1=0$ since it's a large tank and velocity is going to be very small at point 1, which also means we could approximate the pressure at point 1 to be what it was in the static situation i.e. 500 kPa + $p_a$.

Therefore, we have $p_1 - p_2= 500 + p_a - p_a = 500$.

Does above explanation makes sense?

 

[haruspex]

That might make sense. The problem statement might not have an error.

The term "water pressure" in the question could mean the pressure due to the water column above it i.e due to $\rho h g$. Using this fact, I have presented my take on this question as below.

The pressure at point 2 was (water pressure + atmospheric pressure) i.e. 500 kPa + $p_a$ before the sudden leak started. Now we know that in a static fluid the pressures at points having the same height of liquid above them are equipressure points; therefore the pressure at point 1 was also 500 kPa + $p_a$ just before the leak.

When the leak suddenly started we have no more a static fluid scenario but a flowing fluid scenario. The pressure at point 2 now changes to atmospheric pressure i.e $p_a$ since it's in contact with atmosphere. Now, at point 1 we are assuming $v_1=0$ since it's a large tank and velocity is going to be very small at point 1, which also means we could approximate the pressure at point 1 to be what it was in the static situation i.e. 500 kPa + $p_a$.

Therefore, we have $p_1 - p_2= 500 + p_a - p_a = 500$.

Does above explanation makes sense?

Yes

 

[vcsharp2003]

Yes

So, it's just a tricky question rather than a badly worded question.

 

[haruspex]

So, it's just a tricky question rather than a badly worded question.

No, it is badly worded. It says "where the pressure is" instead of "where the pressure had been".

 

[vcsharp2003]

No, it is badly worded. It says "where the pressure is" instead of "where the pressure had been".

Yes, it could have been more precise. Even though, one can say that 500 kPa could not be the pressure at 2 after leak starts since then it's obviously 1 atmosphere. So, 500 kPa must be the water pressure before leak started.

But I agree, it could have been more precise in its statement rather than leaving it to the student to decide.

 

[256bits]

But it says water pressure at 2 is 500 kPa and not at 2, unless I'm reading it wrong.

The problem has indeterminate sentence phrasing.
The comma after the part "... in fig 14-2, ".
Apparantly the author thought everyone would clearly see the phase "where the pressure is 500 kPa." relates back to the tank.

He just could have wrote,
" A water tank, where the water pressure is 500kPa, springs a leak at position 2."

Or better yet,
Where the water pressure is 500kPa, a tank springs a leak.