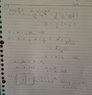Calculating R, L, and Optimal C for e(t) and i(t) in a Power Circuit

  • Thread starter MissP.25_5
  • Start date
  • Tags
    Capacitance
In summary, To find the values of resistance R and inductance L, you can use the given equations for power source voltage and current. To find the capacitance value of the capacitor C that maximizes the magnitude of the phasor voltage V, you can maximize the difference between the voltages V1 and V2, which are functions of C and the power source voltage E. The current phasor through the 25 ohm resistor and C can be calculated using E and the impedance Z, which is a function of C. Finally, you can use the derivative of the voltage difference function to determine the value of C that maximizes the magnitude of V.
  • #1
MissP.25_5
331
0
I have done no. 1, but I don't how to do no. 2. Someone, please help.

Power source voltage e(t) = sqrt(2)*20sin2t [V],
current i(t) = 5sin(2t - pi/4) [A].

1. Find the values of resistance R, and inductance L.
2. Let V be the phasor of voltage v(t). Find the capacitance value of the capacitor C that maximizes the magnitude of V.
 

Attachments

  • FIND C.jpg
    FIND C.jpg
    24.6 KB · Views: 397
  • IMG_5838.jpg
    IMG_5838.jpg
    25.1 KB · Views: 438
Physics news on Phys.org
  • #2
You know R and L so you can come up with the voltage V1 between L and R.

You can also write an expression for the voltage V2 between the 25 ohm resistor and C. This will of course be a function of E and C.

Then maximize (V2 - V1) with respect to C. (The value of C will not be a function of E).
All V are phasors. E is the phasor of e(t).
 
  • #3
rude man said:
You know R and L so you can come up with the voltage V1 between L and R.

You can also write an expression for the voltage V2 between the 25 ohm resistor and C. This will of course be a function of E and C.

Then maximize (V2 - V1) with respect to C. (The value of C will not be a function of E).
All V are phasors. E is the phasor of e(t).

How do I write an expression for the voltage V2 between the 25 ohm resistor and C? I don't know the current across it.
 
  • #4
MissP.25_5 said:
How do I write an expression for the voltage V2 between the 25 ohm resistor and C? I don't know the current across it.

The current phasor through (not "across") C and the 25 ohm is E/Z where Z = 25 -j/ωC.
 
  • #5
rude man said:
The current phasor through (not "across") C and the 25 ohm is E/Z where Z = 25 -j/ωC.

So now I get V1-V2. Next, do I write the function of d(v1-v2)/dC = 0?
 
  • #6
rude man said:
The current phasor through (not "across") C and the 25 ohm is E/Z where Z = 25 -j/ωC.

Can you check this, please? Is this correct?
 

Attachments

  • part 1.jpg
    part 1.jpg
    27 KB · Views: 355
  • part2.jpg
    part2.jpg
    18.8 KB · Views: 373
Last edited:
  • #7
MissP.25_5 said:
So now I get V1-V2. Next, do I write the function of d(v1-v2)/dC = 0?

Absolutely right! You might want to make sure it's a maximum, not a minimum or inflection point).
 
  • #8
MissP.25_5 said:
Can you check this, please? Is this correct?

Sorry, that would go a bit beyond what we're supposed to do.
 

FAQ: Calculating R, L, and Optimal C for e(t) and i(t) in a Power Circuit

How do I calculate the resistance (R) in a power circuit?

To calculate the resistance in a power circuit, you will need to use Ohm's law which states that resistance (R) is equal to the voltage (V) divided by the current (I). This can be represented by the equation R = V/I. Make sure to use the appropriate units of measurement for voltage (volts) and current (amps) to get the correct value for resistance.

What is the formula for calculating inductance (L) in a power circuit?

The formula for calculating inductance in a power circuit is L = V/(2 * pi * f * I), where V is the voltage, f is the frequency, and I is the current. This formula is based on the relationship between voltage and current in an inductor, which is represented by the equation V = L * (dI/dt), where dI/dt is the rate of change of current over time.

How do I determine the optimal capacitance (C) for a power circuit?

The optimal capacitance for a power circuit can be determined by first calculating the reactance of the circuit using the formula Xc = 1/(2 * pi * f * C), where f is the frequency and C is the capacitance. Then, you can use the formula Z = sqrt(R^2 + Xc^2) to calculate the impedance of the circuit. The optimal capacitance will be equal to the value of C that results in the lowest impedance value.

Is there a specific order in which I should calculate R, L, and C in a power circuit?

There is no specific order in which you should calculate R, L, and C in a power circuit. However, it may be helpful to first determine the values for R and L before calculating the optimal C, as the values for R and L can affect the optimal C value. It is also important to double check your calculations and ensure that all units of measurement are consistent.

How can I verify the accuracy of my calculations for R, L, and C in a power circuit?

To verify the accuracy of your calculations, you can use a multimeter to measure the voltage and current in the circuit and compare them to the values you calculated. If there are any discrepancies, double check your calculations and make sure all units of measurement are consistent. You can also use simulation software to model the circuit and compare the results to your calculations.

Similar threads

Resistance, Inductance and Capacitor
Replies
1
Views
1K
Engineering Intro to Power Systems: AC RLC circuit voltage calculations
Replies
17
Views
2K
Current flowing through resistor in RLC circuit (C in parallel with L)
Replies
19
Views
2K
Engineering Open Modelica, work with an RLC-circuit
Replies
1
Views
2K
Engineering Intro to Power Systems: Calculating Power
Replies
7
Views
1K
Engineering Finding Time Constant T Without L for an LR Circuit
Replies
2
Views
1K
Engineering AC Source driving an RLC circuit....
Replies
5
Views
2K
Calculation of current in driven series RLC circuit
Replies
8
Views
850
Engineering Calculating Capacitive Reactance
Replies
21
Views
2K
Engineering Circuit theory: capacitor energy storage and discharging/charging times?
Replies
10
Views
2K

Hot Threads

Recent Insights

Back
Top