Calculating Shannon Entropy of DNA Sequences

[GravityX]

Homework Statement

Calculate the information content of a DNA base pair

Relevant Equations

$I(A)=-\sum\limits_{x=A}^{}P_xlog_2(P_x)$

Unfortunately, I have problems with the following task

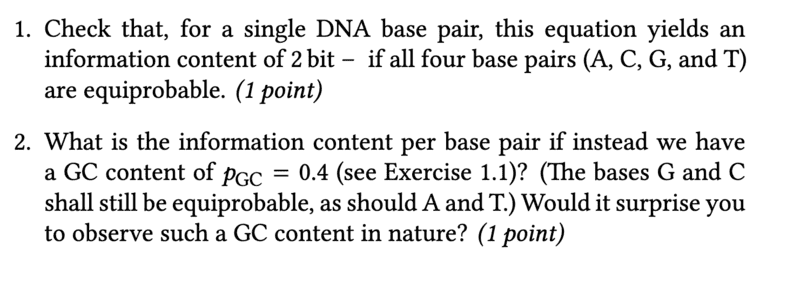
For task 1, I proceeded as follows. Since the four bases have the same probability, this is $P=\frac{1}{4}$ I then simply used this probability in the formula for the Shannon entropy:

$$I=-\frac{1}{4}log_2(\frac{1}{4})-\frac{1}{4}log_2(\frac{1}{4})-\frac{1}{4}log_2(\frac{1}{4})-\frac{1}{4}log_2(\frac{1}{4})=2$$

Unfortunately, I am not quite sure about task 2, but a GC content indicates how high the proportion of GC is in the DNA, so it means that AT must be present at 60 % and GC at 40 %. Is the calculation then as follows:

$$I=-0.4log_2(0.4)-0.4log_2(0.4)-0.6log_2(0.6)-0.6log_2(0.6)=1.94$$

 

[Steve4Physics]

Unfortunately, I am not quite sure about task 2, but a GC content indicates how high the proportion of GC is in the DNA, so it means that AT must be present at 60 % and GC at 40 %. Is the calculation then as follows:$$I=-0.4log_2(0.4)-0.4log_2(0.4)-0.6log_2(0.6)-0.6log_2(0.6)=1.94$$

Not familiar with the topic but if the formula in 'Relevant Equations' is correct then, for task 2, the four probabilties should presumably be:
P(G) = 0.2 (i.e. not 0.4)
P(C) = 0.2 (i.e. not 0.4)
P(A) = 0.3 (i.e. not 0.6)
P(T) = 0.3 (i.e. not 0.6)
(They have to add-up to 1.)

 

[haruspex]

Not sure I understand the first question. It lists A, C, G and T as the four "base pairs". No, they are bases, the pairs being GC, AT.
But treating it as a matter of base pairs would yield $-\frac 12\ln(\frac 12)-\frac 12\ln(\frac 12)=1$.
The question ought to read "for a single DNA base" (i.e., per base in a single strand).

This may be what fooled you into using 0.4 and 0.6 instead of 0.2 and 0.3 in the second question. But note that we can only get 0.2 and 0.3 by assuming that the orientations of the base pairs (which base is in which strand) are independent. One could imagine some sort of autocorrelation instead.

As to whether it would be surprising, that might depend whether we consider also the relative stabilities of the base pairs and the scheme that maps codons to amino acids.

 

[Steve4Physics]

Not sure I understand the first question. It lists A, C, G and T as the four "base pairs". No, they are bases, the pairs being GC, AT.

By convention, when writing base-pair sequences, to be concise we use a single letter for each base pair. The letter is the base present on the ‘forward’ strand. See link below.

With this convention:
‘A’ represents adenine-thymine on the double strand.
‘C’ represents cytosine-guanine on the double strand.
‘G’ represents guanine- cytosine on the double strand.
‘T’ represents thymine- adenine on the double strand.

For example (using lower case for the bases) the sequence TATAGC represents the double strand: tatagc atatcg
From https://www.futurelearn.com/info/courses/bacterial-genomes-bioinformatics/0/steps/47002:
“Despite being a double helix of complementary DNA sequences, DNA is almost always represented as a single sequence.”

 

[haruspex]

By convention, when writing base-pair sequences, to be concise we use a single letter for each base pair. The letter is the base present on the ‘forward’ strand.

Ok, thanks.

 

[GravityX]

Thank you Steve4Physics and haruspex for your help , I had completely forgotten that the 0.4 was for the pair and not one base alone.