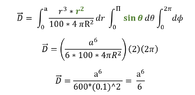Calculating the charge if the electric field density = 0

[falyusuf]

Homework Statement

Attached below.

Relevant Equations

Attached below.

Question:

Relevant Equations:

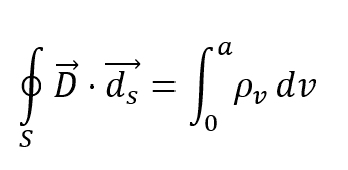
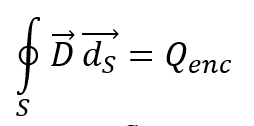
My attempt:

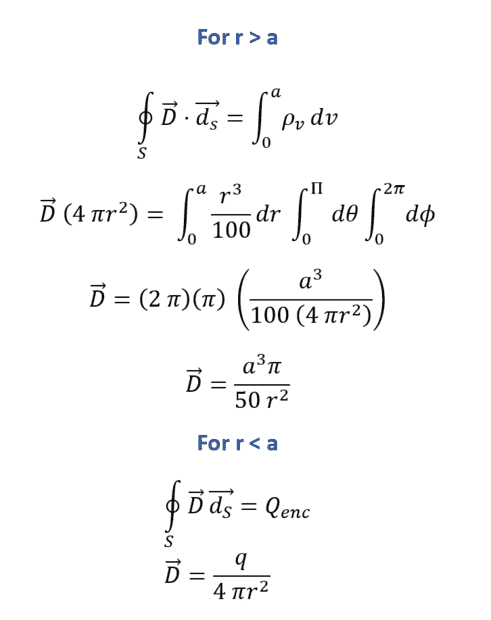
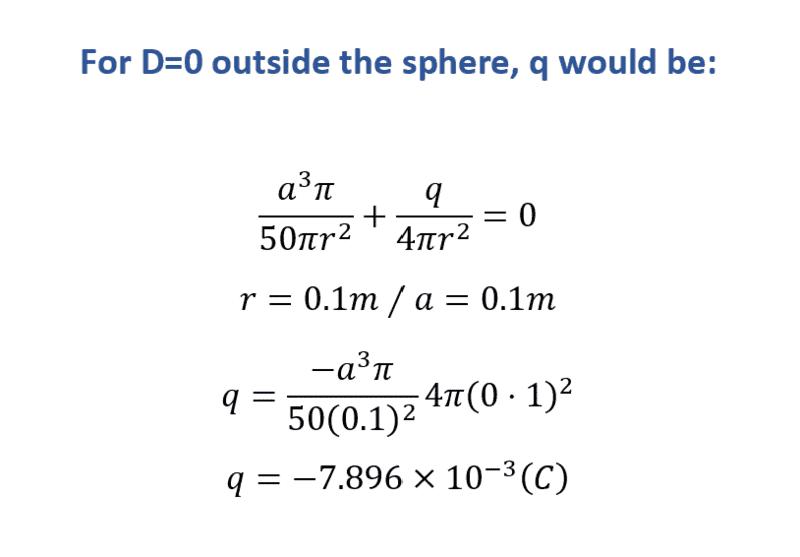
Could someone please confirm my solution?

 

[mjc123]

Doesn't look right.
1. Is r a variable or a constant?
2. Is dv = drdθdφ?
3. Is the integral of r³/100 = a³/100?

 

[falyusuf]

1. Is r a variable or a constant?

r is constant as it is the radius of the sphere whereas a is variable.

2. Is dv = drdθdφ?

yes, (for the sphere)

3. Is the integral of r3/100 = a3/100?

I was mistaken here, it should be a^4 / 400

 

[mjc123]

r is constant as it is the radius of the sphere whereas a is variable.

You are integrating by dr from limits 0 to a, yet treat 4πr² as a constant.

yes, (for the sphere)

Really? What are the dimensions on either side?

 

[falyusuf]

You are integrating by dr from limits 0 to a, yet treat 4πr² as a constant.

Really? What are the dimensions on either side?

Is dv = r^2 sin theta dtheta dPhi dr ?
I tried to correct my mistakes and this is what I got:

 

[mjc123]

I still think you're using r in a confusing way. Use r for the integration variable and R (constant) for the distance at which you want to evaluate the field. Then you are not integrating r³, but r⁵/R².

 

[falyusuf]

I still think you're using r in a confusing way. Use r for the integration variable and R (constant) for the distance at which you want to evaluate the field. Then you are not integrating r³, but r⁵/R².

Am I right now?

 

[mjc123]

The integration variable is r, not R. And the upper limit is a, not R.

 

[falyusuf]

The integration variable is r, not R. And the upper limit is a, not R.

Sorry I was confused.

Right?

 

[mjc123]

R is not 0.1 m. a is 0.1 m. You should have R² in the denominator for both expressions, and it will cancel out.
You need to be very careful in distinguishing the various distances and using consistent notation for them. Too many rs is a recipe for confusion.

 

[falyusuf]

R is not 0.1 m. a is 0.1 m. You should have R² in the denominator for both expressions, and it will cancel out.
You need to be very careful in distinguishing the various distances and using consistent notation for them. Too many rs is a recipe for confusion.

Thanks for clarifying. I solved it using two methods and I got the same magnitude with opposite sign. Could you please figure out my mistake?

 

[mjc123]

I'm not quite sure what you're doing in method 2, but the point charge at the centre should be equal to minus the charge obtained by integrating ρ over the volume of the sphere. So from outside it behaves like a point charge of zero.
I think your answer of -2.09e-8 C is correct. (I did it in my head and got -2.09e-4 C; I think I must have accidentally switched the factor of 100 from the bottom to the top:).)

 

[falyusuf]

I'm not quite sure what you're doing in method 2, but the point charge at the centre should be equal to minus the charge obtained by integrating ρ over the volume of the sphere. So from outside it behaves like a point charge of zero.
I think your answer of -2.09e-8 C is correct. (I did it in my head and got -2.09e-4 C; I think I must have accidentally switched the factor of 100 from the bottom to the top:).)

I got it now.. Thank you so much. Appreciate your help.