Amaelle
- 309
- 54
- Homework Statement
- look at the image
- Relevant Equations
- stocks theorem
Greetings
The exercice consist of calulating the circuitation of the Field F along a the borders of the region omega
my problem was how they found that y goes from 0 to h ( for 0 it´s clear but the mystery for me is h)
Thank you!
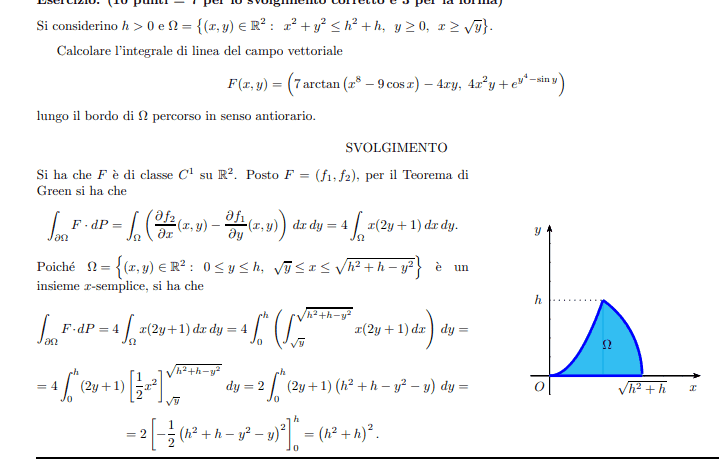
The exercice consist of calulating the circuitation of the Field F along a the borders of the region omega
my problem was how they found that y goes from 0 to h ( for 0 it´s clear but the mystery for me is h)
Thank you!