- #1
grandpa2390
- 474
- 14
Homework Statement
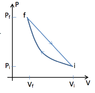
I am trying to calculate the efficiency of this heat engine that has two step. an adiabatic compression, followed by a linear expansion back to the original point. I keep getting an efficiency of 1, which I know can't be right...
Homework Equations
##e = \frac{W}{Q_H}##
The Attempt at a Solution
Is the W the net Work or the Work done by the gas. (I am guessing the Work done by the gas)
and Q_H is the heat absorbed. which is 0 for the adiabatic process. and since Q=0 for an adiabatic process, then Q_H is equal to the negative of the net work.
this is giving me a efficiency greater than 1 though.
Last edited: