Calculating torque at a distance from the lever

In summary, the speaker is an engineering student who is struggling with calculating the overall moments of a motorcycle's rear end. They have no problem with the first two moments, but are struggling with the third. They have provided a diagram and an equation they found through research, but they do not fully understand how it works. They are seeking help in understanding how to calculate the torque in this system.
  • #1
Ross Hanna
2
0
Hi there, I am an engineering student studying my masters, but have run into a roadblock in my calculations. I know I'm missing something obvious but i just can't see it. Too close to the woods and all that!

Anyway, i have the rear end of a motorcycle. to calculatew the overall moments, i have to find the three individual moments acting on it. the first two I'm fine with, but the third I'm struggling.

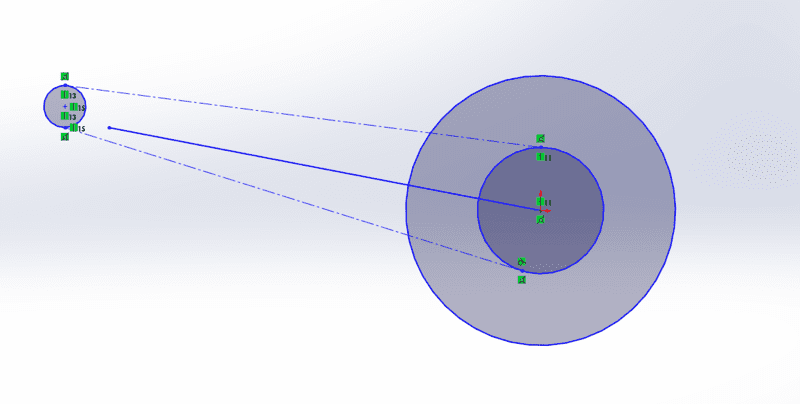
I have a rear end of a motorcycle. The wheel has a sprocket/ gear rigidly attached. A chain connects this gear to another at a distance Lc centre to centre and angle eta with a tension T. Lc is constructed of a triangle with sides a (horizontal) and b (vertical). The wheel is attached to a swingarm with length L, and angle to the horizontal of phi. The gears have radius Rc and Rs. The front sprocket rotates at V rad/s and transmits a power P.

I have attached a diagram to help.
Rear Swingarm.PNG
From research, the moment should be;

T*(Rc - L*sin(phi-eta))

I can get the equation by working backwards, but i don't understand how it works. The basics physics question is this. If i have a system such as below, how do i calculate the torque?

Torque Problem.jpg


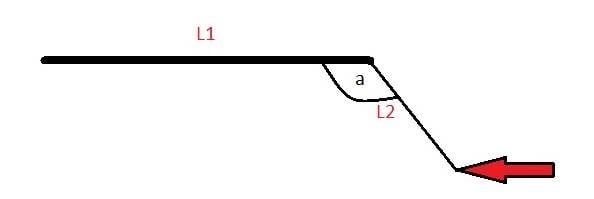
if the torque was applid to L1, i would just use T = F.r.sin(theta), but I'm unsure how to resolve the forces for this.

Thanks for any help

Ross
 

Attachments

  • Rear Swingarm.PNG
    Rear Swingarm.PNG
    28.4 KB · Views: 547
  • Torque Problem.jpg
    Torque Problem.jpg
    4.1 KB · Views: 555
Engineering news on Phys.org
  • #2
When you say you're trying to calculate "the overall moments" what are you referring to?

I'm kind of assuming the speed/acceleration of the driving wheel, in which case the suspension geometry doesn't play into the calculation at all. Just compare the ratio or radii between the two sprockets for the torque multiplication, and apply a reasonable efficiency factor for power loss through the chain drive.

Am I missing something that you're trying to calculate?
 

Related to Calculating torque at a distance from the lever

1. How do you calculate torque at a distance from the lever?

To calculate torque at a distance from the lever, you need to multiply the force applied to the lever by the distance from the pivot point (or fulcrum). This is represented by the equation T = F * d, where T is torque, F is force, and d is distance.

2. What is the unit of measurement for torque?

Torque is typically measured in Newton-meters (N*m) or foot-pounds (ft*lbs), depending on the unit system being used.

3. How does the distance from the lever affect the torque?

The distance from the lever to the pivot point, also known as the moment arm, directly affects the torque. The longer the distance, the greater the torque, and vice versa.

4. Can torque be positive or negative?

Yes, torque can be positive or negative depending on the direction of the force being applied. If the force is applied in a clockwise direction, the torque is considered negative, and if it is applied in a counterclockwise direction, the torque is positive.

5. How is torque at a distance from the lever used in real-world applications?

Torque at a distance from the lever is used in many real-world applications, such as using a wrench to loosen or tighten bolts, using a lever to lift heavy objects, and using a crank to rotate machinery. It is also an important concept in understanding the mechanics of rotation and how forces act on objects.

Similar threads

Convert moment force at lever support
    • Mechanical Engineering
Replies
1
Views
1K
Understanding and Troubleshooting Handlebar Load Calculations
    • Mechanical Engineering
Replies
28
Views
961
How to calculate required torque for a robot arm
    • Mechanical Engineering
Replies
2
Views
2K
What is the force generated by a lever in a closed position?
    • Mechanical Engineering
Replies
7
Views
1K
The effect of slightly slanted distance (arm) on torque output
    • Mechanical Engineering
Replies
4
Views
1K
Minimizing the friction of the end of a lever against the top of a moving piston
    • Mechanical Engineering
Replies
21
Views
1K
Torque calculation for a new loading mechanism
    • Mechanical Engineering
Replies
8
Views
1K
How much should I adjust torque when using anti-seize?
    • Mechanical Engineering
Replies
21
Views
736
Automotive Need help calculating load torque and acceleration torque of a vehicle
    • Mechanical Engineering
Replies
8
Views
4K
Calculating Effort Needed for Unevenly-Loaded Class 2 Lever
    • Mechanical Engineering
Replies
8
Views
3K

Hot Threads

Recent Insights

Back
Top