Ripcrow
- 82
- 7
- TL;DR Summary
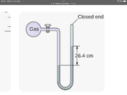
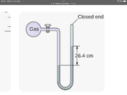
- I have built a purpose designed pump. I have a sealed container filled with ambient temperature water and have attached a tube to act as a manometer (water ) One end is attached to the water container and the other end is blocked off.
Should be a simple test to see how much vacuum can be created by the pump but it is lifting the water in the manometer over 40 inches on one side. From what I’ve seen when reading a manometer you have to double the length on one side. But this means it’s achieving 80 inches h2o which is impossible also from what I’ve read.
The manometer maxes out at 40 inches and it will continue to pull the water into the container so I’m not sure where the actual maximum is but the numbers don’t seem right either.
The other variable is I’m using a flexible tube as the manometer so it does squash down which would account for some variation. The container I’m pumping from holds about 2 litres of water and the manometer holds about 400 millilitres. Am I reading the manometer correctly?
The pump was designed to create a strong vacuum but the numbers are supposedly impossible.
The manometer maxes out at 40 inches and it will continue to pull the water into the container so I’m not sure where the actual maximum is but the numbers don’t seem right either.
The other variable is I’m using a flexible tube as the manometer so it does squash down which would account for some variation. The container I’m pumping from holds about 2 litres of water and the manometer holds about 400 millilitres. Am I reading the manometer correctly?
The pump was designed to create a strong vacuum but the numbers are supposedly impossible.
Last edited by a moderator: