Calculation of Thomas Precession in Rindler's relativity book

[NoobieDoobie]

Homework Statement

A problem from Rindller's relativity book (Check below)

Relevant Equations

Lorentz Transformations

I am trying to work through rindler's relativity book. However, I got stuck at what I think should be a simple approximation.

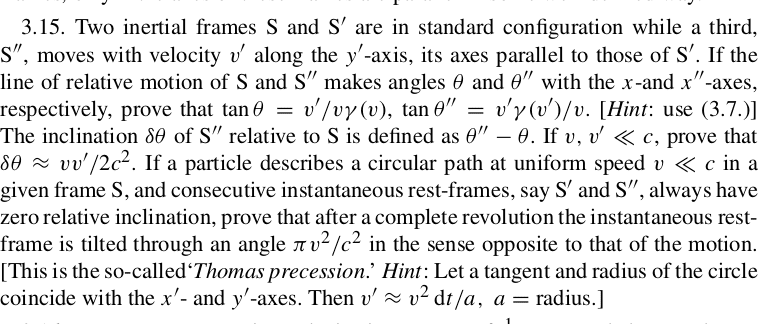
I calculated the angles $\theta$ and $\theta''$ using the formula for velocity transformation. If I simply expand $\gamma(v)$ and $\gamma(v')$ using taylor series and calculate $tan(\theta'')-tan(\theta) = \frac{v'}{2vc^2}(v^2+v'^2)$. First term of this formula is precisely the answer. However, I would have to calculate $tan(\theta''-\theta)$, invert the equation and approximate again. This doesn't seem to be what rindler wants us to do though. There must be a simpler way to derive the formula. What am I doing wrong?

Edit : If I assume $v' << v$, I do get the same formula as rindler. I checked from Goldstein's book and he derives the same formula under the assumption $v'<<v$. I am wondering if Rindler might just have forgotten to add it in the problem statement?

 

[TSny]

Edit : If I assume $v' << v$, I do get the same formula as rindler. I checked from Goldstein's book and he derives the same formula under the assumption $v'<<v$. I am wondering if Rindler might just have forgotten to add it in the problem statement?

Yes, you are right. The assumption $v' << v$ should have been stated by Rindler.

 

[NoobieDoobie]

Yes, you are right. The assumption $v' << v$ should have been stated by Rindler.

I'm not sure if it's necessary. I found exact formula for wigner rotation under two perpendicular boosts which turns out to be $tan(\omega) == -\frac{\gamma_2\gamma_1\beta_2\beta_1}{\gamma_2+\gamma_1}$. Which gives the same result as Rindler under the limits $\beta_1, \beta_2 << 1$. So we don't need to require $\beta_2<<\beta_1$ additionally.

 

[TSny]

I'm not sure if it's necessary. I found exact formula for wigner rotation under two perpendicular boosts which turns out to be $tan(\omega) == -\frac{\gamma_2\gamma_1\beta_2\beta_1}{\gamma_2+\gamma_1}$. Which gives the same result as Rindler under the limits $\beta_1, \beta_2 << 1$. So we don't need to require $\beta_2<<\beta_1$ additionally.

Oh, that's good! And it surprised me. But, I was able to verify $\tan(\omega) = \large-\frac{\gamma_2\gamma_1\beta_2\beta_1}{\gamma_2+\gamma_1}$ after some manipulations using the trig identity $$\tan(\alpha-\beta) = \frac{\tan \alpha - \tan \beta}{1+\tan \alpha \tan \beta}$$.
I should have had more faith in Rindler!

 

[NoobieDoobie]

Oh, that's good! And it surprised me. But, I was able to verify $\tan(\omega) = \large-\frac{\gamma_2\gamma_1\beta_2\beta_1}{\gamma_2+\gamma_1}$ after some manipulations using the trig identity $$\tan(\alpha-\beta) = \frac{\tan \alpha - \tan \beta}{1+\tan \alpha \tan \beta}$$.
I should have had more faith in Rindler!

That means I must be doing some trivial calculation mistake since I used same trig identity but my result doesn't match with $\tan(\omega)$.

 

[TSny]

That means I must be doing some trivial calculation mistake since I used same trig identity but my result doesn't match with $\tan(\omega)$.

Here's an outline of my calculation, which might not be the best.

We know $\tan \theta'' = \large \frac{\beta \,' \gamma'}{\beta}$ and $\tan \theta = \large \frac{\beta \,'}{\beta \gamma }$.

Here, $\gamma = \large \frac 1 {\sqrt{1-\beta^2}}$ and $\gamma' = \large \frac 1 {\sqrt{1-\beta \,'^2}}$.

The tangent function trig identity is then $$\tan(\theta'' - \theta) = \frac{\beta \,'}{\beta} \frac {\gamma' - \frac 1 {\gamma}}{1+(\frac{\beta \,'^2}{\beta^2})\frac{\gamma'}{\gamma}} = \beta \beta \,' \frac{\gamma' \gamma - 1}{\beta^2\gamma + \beta \,'^2 \gamma'}$$
It's easy to show $\beta^2 \gamma = \gamma - \frac 1 {\gamma}$ and $\beta \,'^2 \gamma' = \gamma' - \frac 1 {\gamma'}$.

So, $$\tan(\theta'' - \theta) = \beta \beta \,' \frac{\gamma' \gamma - 1}{\gamma - 1/\gamma + \gamma' - 1/\gamma'}$$
Simplify.

 

[NoobieDoobie]

When I had tried it, I first stared at the expression for $\tan(\omega)$ and concluded there's no way it can be as simple as the answer we're looking for. Now I understand why faith plays an important role.

Thanks for the help!