Can AC Be Less Than Twice FG in a Square Geometry Setup?

[anemone]

Here is this week's POTW:

-----

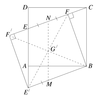
Let $ABCD$ be a square, and let $E$ be an internal point on side $AD$. Let $F$ be the foot of the perpendicular from $B$ to $CE$. Suppose $G$ is a point such that $BG = FG$, and the line through $G$ parallel to $BC$ passes through the midpoint of $EF$. Prove that $AC<2\cdot FG$.

-----

Remember to read the https://mathhelpboards.com/showthread.php?772-Problem-of-the-Week-%28POTW%29-Procedure-and-Guidelines to find out how to https://mathhelpboards.com/forms.php?do=form&fid=2!

 

[anemone]

No one answered last week's problem, (Sadface) but you can find the suggested solution as shown below:

Spoiler

View attachment 8747

Note that for given $E$ and $F$, there is only one point $G$ with the required properties.

Since $BG=FG$, $G$ must lie on the perpendicular bisector of $BF$, and by the second condition, $G$ lies on the line through the midpoint of $EF$ parallel to $BC$.

$G$ must thus be the unique intersection of these two lines. We now give an alternative construction for $G$ from which the result will follow.

Since $BA$ and $CD$ are parallel and equal, we may translate $\triangle CDE$ to give a triangle $\triangle BAE'$. Then $E',\,A,\,E$ are collinear, with $E'E=E'A+AE=ED+AE=AD=BC$ and $E'E,\,BC$ are parallel, so we may also translate $\triangle BCF$ to give $\triangle E'EF'$. Then $F,\,E,\,F'$ are collinear. Now, $E'F'=BF$, and $\angle E'F'E=\angle BFC=90^\circ=\angle BFE$. Hence, $BE'F'F$ is a rectangle.

Let $G'$ be its center. Certainly $BG'=FG'$. Let the line through $G'$ parallel to $BC$ hit $BE',\,FF'$ at $M,\,N$ respectively, then symmetry gives $E'M=FN$. However, by translation, $EN=E'M$, so $EN=FN$, and the parallel to $BC$ through $G'$ bisects $EF$. Thus, by the uniqueness of $G$ already proven, $G=G'$, the center of $BE'F'F$.Now, by AM-GM (strict because $BF<BC=CD<CE=BE'$,

$\begin{align*}2\cdot FG&=FE'\\&=\sqrt{BF^2+BE^2}\\&>\sqrt{2\cdot BF\cdot BE'}\\&=\sqrt{2[BFF'E']}\\&=\sqrt{2([BAE']+[E'EF']+[ABFE])}\,\,\,\text{here brackets denote areas}\\&=\sqrt{2([CDE]+[BCF]+[ABFE])}\\&=\sqrt{2[ABCD]}\\&=\sqrt{2}\cdot AB\\&=AC\,\,\,\text{(Q.E.D.)}\end{align*}$