- #1
nil1996
- 301
- 7
Rolle's theorem:
Statements:
If y =f(x) is a real valued function of a real variable such that:
1) f(x) is continuous on [a,b]
2) f(x) is differentiable on (a,b)
3) f(a) = f(b)
then there exists a real number c[itex]\in[/itex](a,b) such that f'(c)=0
what if the the f(x) is like the following graph:
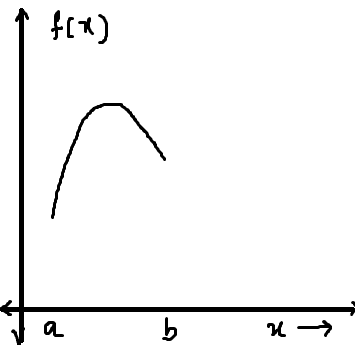
here there is a point 'c' for which f'(c) =0 but f(a) [itex]\neq[/itex] f(b)
So to take such cases in consideration can we make a change to the last statement of Rolle's theorem as:
3)f(c) > [f(a),f(b)] Or f(c)<[f(a),f(b)]
are there any exceptions to the above statement?
Statements:
If y =f(x) is a real valued function of a real variable such that:
1) f(x) is continuous on [a,b]
2) f(x) is differentiable on (a,b)
3) f(a) = f(b)
then there exists a real number c[itex]\in[/itex](a,b) such that f'(c)=0
what if the the f(x) is like the following graph:
here there is a point 'c' for which f'(c) =0 but f(a) [itex]\neq[/itex] f(b)
So to take such cases in consideration can we make a change to the last statement of Rolle's theorem as:
3)f(c) > [f(a),f(b)] Or f(c)<[f(a),f(b)]
are there any exceptions to the above statement?
Attachments
Last edited: