Can you solve this challenging integral using a clever modification?

[omri_mar]

hi guys!
can you please help me with this integral? I've tried some subs but i really don't know how to start...
Thank you !

 

[mfb]

Expanding with $\sqrt{\ln(9-x)}-\sqrt{\ln(3+x)}$ gives
$$\frac {\sqrt{\ln(9-x)}\left(\sqrt{\ln(9-x)}-\sqrt{\ln(3+x)}\right)} {\ln(9-x)-\ln(3+x)}$$

That can be split into two parts, where one part looks easy and the other part can be simplified significantly. I don't know if that will lead to a solution, however.

 

[omri_mar]

first of all thank you!
Ive tried this and it lead me nowhere..

 

[mfb]

Hmm okay, I don't see how to solve the remaining part either.

An interesting observation: 3+x goes from 5 to 7, while 9-x goes from 7 to 5. There could be some symmetry to exploit, but I did not find it yet.

 

[omri_mar]

Thats actually very interesting because according to the answers the answer is 1.
Can someone help me...?

 

[Curious3141]

Hint: substitute $\displaystyle x = 6-y$.

EDIT: numerous glitches in the PF software are making it difficult to post. Basically, I wanted to add: don't try to simplify the integrand, etc. Just do the sub, and see what happens to the bounds. This is actually a very simple problem in disguise.

 

[omri_mar]

I can't remove the disguise... :(

 

[omri_mar]

this is what i get

 

[mfb]

Ah nice, that is the right way to solve it.
@omri_mar: You can modify this new integrand to look similar to the old one now.

 

[omri_mar]

I don't get it. How can I continue?

 

[cpt_carrot]

Try adding together the new integral and the old one

 

[omri_mar]

what do you mean?

 

[mfb]

You have an equation (old integral)=(new integral). Try to write (new integral) as (something)-(old integral). This gives you an equation like 2*(old integral)=(something), and (something) is easy to calculate.

Don't write PMs, please.

 

[Curious3141]

this is what i get

Sorry for the late reply - I just noticed your bounds are off in the attachment to post 8. Your bounds should be reversed (identical to the original integral). Don't forget that $dy = -dx$. Other than that, the other posters have already told you how to proceed. Remember that in a definite integral, the variable of integration is basically just a dummy variable, so whether it's x or y, it doesn't matter. So just replace the y in the new integral with x, and add it to the original integral. Now you have twice the original integral, and it's equal to something very simple.

 

[SammyS]

this is what i get

(Most of post deleted in Edit. It was pretty much redundant with Curious3141 post. )

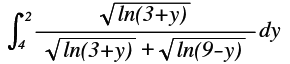
Now modify the numerator by adding and subtracting ... (something clever) .