Capacitor exercise -- Calculate the force needed to withdraw the dielectric

[Jack99123]

Homework Statement

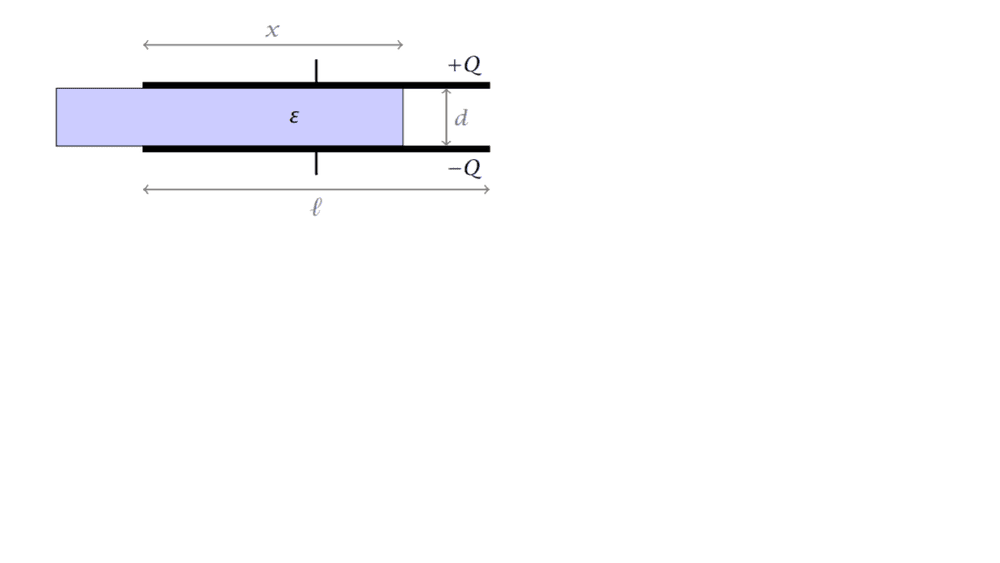
Between two conducting plates (length $l$, width $w$ and distance $d$) there is an insulator (permittivity \epsilon). The capacitor is charged with voltage $V$ (charge $Q$). After this the voltage source is removed. The insulator is moved to the left in the direction of $l$ a distance $l-x$. Calculate the force that the electric field tries to drag the insulator back
to between the conducting plates. (Hint. F=-∇U, expression for potential energy with
capacitance, the capacitor charge is constant, voltage changes)

Relevant Equations

$C_e=C_1+C_2$
$U=\frac{1}{2}*C*V^2$
$C=\epsilon \frac{A}{d}$
$\vec F =-\nabla U$

First, I think that I need to calculate the capacitance. It is $C=\epsilon_0*\frac{l*w}{d}-x*\frac{w*\epsilon_0}{d}+\epsilon*\frac{x*w}{d}$. After that I should calculate the potential energy. It is $U=\frac{1}{2}*C*V^2$. After that I should take its gradient to get the force. So $\vec F =-\nabla U=-\frac{d}{dx}U*\vec i=-\frac{(\frac{-w*\epsilon_0}{d}+\frac{\epsilon*w}{d})*v^2}{2}*\vec i$
. Is this correct and if it's not, could you please help me?

 

[Steve4Physics]

First, I think that I need to calculate the capacitance. It is $C=\epsilon_0*\frac{l*w}{d}-x*\frac{w*\epsilon_0}{d}+\epsilon*\frac{x*w}{d}$. After that I should calculate the potential energy. It is $U=\frac{1}{2}*C*V^2$. After that I should take its gradient to get the force. So $\vec F =-\nabla U=-\frac{d}{dx}U*\vec i=-\frac{(\frac{-w*\epsilon_0}{d}+\frac{\epsilon*w}{d})*v^2}{2}*\vec i$
. Is this correct and if it's not, could you please help me?View attachment 280047

Your overall strategy looks OK but note:

1) You can’t have voltage (V) in your function for U(x) . (Why not?) You need to express U(x) using only x and fixed values. What quantity remains fixed when the insulator (dielectric) is moved?

2) Your equation:
$C=\epsilon_0*\frac{l*w}{d}-x*\frac{w*\epsilon_0}{d}+\epsilon*\frac{x*w}{d}$
looks correct, but for clarity, note that you have two parallel capacitors, one with area $xw$ and the other with area $(l-x)w$. A clearer expression for C (equivalent to yours) would be:
$C=\frac{\epsilon_0(l-x)w}{d}+\frac{\epsilon xw}{d}$

 

[Jack99123]

So then $Q$ remains constant. I need to use equation $U=\frac{Q^2}{2*C}$. Then I need to use $\vec F=-\nabla U$ which is $\vec i *\frac{Q^2*d*(\epsilon-\epsilon_0)}{2*(\epsilon_0*(l-x)+\epsilon *x)^2*w}$ Is this right?

 

[Steve4Physics]

So then $Q$ remains constant. I need to use equation $U=\frac{Q^2}{2*C}$. Then I need to use $\vec F=-\nabla U$ which is $\vec i *\frac{Q^2*d*(\epsilon-\epsilon_0)}{2*(\epsilon_0*(l-x)+\epsilon *x)^2*w}$ Is this right?

Looks probably OK - but I'm not checking the algebra for you!

Check if the question requires an answer in terms of the initial voltage (V). If so, you will need replace Q with a suitable expression.