- #1
Saitama
- 4,243
- 93
Homework Statement
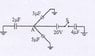
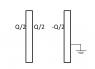
Three capacitors of 2μF, 3μF and 5μF are independently charged with batteries of emf's 5V, 20V and 10V respectively. After disconnecting from voltage sources, these capacitors are connected as shown in figure with their positive polarity connected to A and negative polarity earthed. Now a battery of 20V and an uncharged capacitor of 4μF capacitance are connected to junction A as shown with a switch S. When switch is closed, find:
a)the potential of the junction A.
b)final charges on all four capacitors.
(Attachment 1)
Homework Equations
The Attempt at a Solution
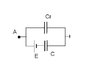
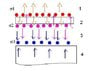
Honestly, I have no clue what happens to the initial charges of capacitors when the switch is closed. I tried to solve the problem as if there are no charges on capacitors initially. Attachment 2 shows the simplified circuit I made. I found the potential at A and got 40/7 V which is incorrect, so I think there is some role of the initial charges which I am unable to figure out. :(
Any help is appreciated. Thanks!